| Family of bifrusta | |
|---|---|
 Example: hexagonal bifrustum Example: hexagonal bifrustum | |
| Faces | 2 n-gons 2n trapezoids |
| Edges | 5n |
| Vertices | 3n |
| Symmetry group | Dnh, , (*n22) |
| Surface area | |
| Volume | |
| Dual polyhedron | Elongated bipyramids |
| Properties | convex |
In geometry, an n-agonal bifrustum is a polyhedron composed of three parallel planes of n-agons, with the middle plane largest and usually the top and bottom congruent.
It can be constructed as two congruent frusta combined across a plane of symmetry, and also as a bipyramid with the two polar vertices truncated.
They are duals to the family of elongated bipyramids.
Formulae
For a regular n-gonal bifrustum with the equatorial polygon sides a, bases sides b and semi-height (half the distance between the planes of bases) h, the lateral surface area Al, total area A and volume V are: and Note that the volume V is twice the volume of a frusta.
Forms
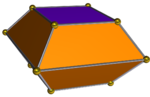
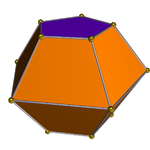
Three bifrusta are duals to three Johnson solids, J14-16. In general, a n-agonal bifrustum has 2n trapezoids, 2 n-agons, and is dual to the elongated dipyramids.
| Triangular bifrustum | Square bifrustum | Pentagonal bifrustum |
|---|---|---|

|
|
|
| 6 trapezoids, 2 triangles. Dual to elongated triangular bipyramid, J14 | 8 trapezoids, 2 squares. Dual to elongated square bipyramid, J15 | 10 trapezoids, 2 pentagons. Dual to elongated pentagonal bipyramid, J16 |
References
- "Octagonal Bifrustum". etc.usf.edu. Retrieved 2022-06-16.
- "Regelmäßiges Bifrustum - Rechner". RECHNERonline (in German). Retrieved 2022-06-30.
- "mathworld pyramidal frustum".
| Convex polyhedra | |||||
|---|---|---|---|---|---|
| Platonic solids (regular) | |||||
| Archimedean solids (semiregular or uniform) | |||||
| Catalan solids (duals of Archimedean) |
| ||||
| Dihedral regular | |||||
| Dihedral uniform |
| ||||
| Dihedral others | |||||
| Degenerate polyhedra are in italics. | |||||
This polyhedron-related article is a stub. You can help Misplaced Pages by expanding it. |


 Note that the volume V is twice the volume of a
Note that the volume V is twice the volume of a