In mathematics, a solid Klein bottle is a three-dimensional topological space (a 3-manifold) whose boundary is the Klein bottle.
It is homeomorphic to the quotient space obtained by gluing the top disk of a cylinder to the bottom disk by a reflection across a diameter of the disk.
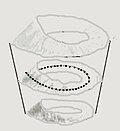
Alternatively, one can visualize the solid Klein bottle as the trivial product , of the möbius strip and an interval . In this model one can see that the core central curve at 1/2 has a regular neighbourhood which is again a trivial cartesian product: and whose boundary is a Klein bottle.
4D Visualization Through a Cylindrical Transformation
One approach to conceptualizing the solid klein bottle in four-dimensional space involves imagining a cylinder, which appears flat to a hypothetical four-dimensional observer. The cylinder possesses distinct "top" and "bottom" four-dimensional surfaces. By introducing a half-twist along the fourth dimension and subsequently merging the ends, the cylinder undergoes a transformation. While the total volume of the object remains unchanged, the resulting structure possesses a singular continuous four-dimensional surface, analogous to the way a Möbius strip has one continuous two-dimensional surface in three-dimensional space, and a regular 2d manifold klein bottle as the boundary.
References
- Carter, J. Scott (1995), How Surfaces Intersect in Space: An Introduction to Topology, K & E series on knots and everything, vol. 2, World Scientific, p. 169, ISBN 9789810220662.
| Manifolds (Glossary, List, Category) | |||||||||
|---|---|---|---|---|---|---|---|---|---|
| Basic concepts | |||||||||
| Main results (list) | |||||||||
| Maps | |||||||||
| Types of manifolds |
| ||||||||
| Tensors |
| ||||||||
| Related | |||||||||
| Generalizations | |||||||||
This topology-related article is a stub. You can help Misplaced Pages by expanding it. |
 to the bottom disk by a
to the bottom disk by a  , of the
, of the  . In this model one can see that
the core central curve at 1/2 has a regular neighbourhood which is again a trivial
. In this model one can see that
the core central curve at 1/2 has a regular neighbourhood which is again a trivial  and whose boundary is a Klein bottle.
and whose boundary is a Klein bottle.