
Johnson–Nyquist noise (thermal noise, Johnson noise, or Nyquist noise) is the electronic noise generated by the thermal agitation of the charge carriers (usually the electrons) inside an electrical conductor at equilibrium, which happens regardless of any applied voltage. Thermal noise is present in all electrical circuits, and in sensitive electronic equipment (such as radio receivers) can drown out weak signals, and can be the limiting factor on sensitivity of electrical measuring instruments. Thermal noise is proportional to absolute temperature, so some sensitive electronic equipment such as radio telescope receivers are cooled to cryogenic temperatures to improve their signal-to-noise ratio. The generic, statistical physical derivation of this noise is called the fluctuation-dissipation theorem, where generalized impedance or generalized susceptibility is used to characterize the medium.

Thermal noise in an ideal resistor is approximately white, meaning that its power spectral density is nearly constant throughout the frequency spectrum (Figure 2). When limited to a finite bandwidth and viewed in the time domain (as sketched in Figure 1), thermal noise has a nearly Gaussian amplitude distribution.
For the general case, this definition applies to charge carriers in any type of conducting medium (e.g. ions in an electrolyte), not just resistors. Thermal noise is distinct from shot noise, which consists of additional current fluctuations that occur when a voltage is applied and a macroscopic current starts to flow.
History of thermal noise
In 1905, in one of Albert Einstein's Annus mirabilis papers the theory of Brownian motion was first solved in terms of thermal fluctuations. The following year, in a second paper about Brownian motion, Einstein suggested that the same phenomena could be applied to derive thermally-agitated currents, but did not carry out the calculation as he considered it to be untestable.
Geertruida de Haas-Lorentz, daughter of Hendrik Lorentz, in her doctoral thesis of 1912, expanded on Einstein stochastic theory and first applied it to the study of electrons, deriving a formula for the mean-squared value of the thermal current.
Walter H. Schottky studied the problem in 1918, while studying thermal noise using Einstein's theories, experimentally discovered another kind of noise, the shot noise.
Frits Zernike working in electrical metrology, found unusual random deflections while working with high-sensitive galvanometers. He rejected the idea that the noise was mechanical, and concluded that it was of thermal nature. In 1927, he introduced the idea of autocorrelations to electrical measurements and calculated the time detection limit. His work coincided with de Haas-Lorentz' prediction.
The same year, working independently without any knowledge of Zernike's work, John B. Johnson working in Bell Labs found the same kind of noise in communication systems, but described it in terms of frequencies. He described his findings to Harry Nyquist, also at Bell Labs, who used principles of thermodynamics and statistical mechanics to explain the results, published in 1928.
Noise of ideal resistors for moderate frequencies

Johnson's experiment (Figure 1) found that the thermal noise from a resistance at kelvin temperature and bandlimited to a frequency band of bandwidth (Figure 3) has a mean square voltage of:
where is the Boltzmann constant (1.380649×10 joules per kelvin). While this equation applies to ideal resistors (i.e. pure resistances without any frequency-dependence) at non-extreme frequency and temperatures, a more accurate general form accounts for complex impedances and quantum effects. Conventional electronics generally operate over a more limited bandwidth, so Johnson's equation is often satisfactory.
Power spectral density
The mean square voltage per hertz of bandwidth is and may be called the power spectral density (Figure 2). Its square root at room temperature (around 300 K) approximates to 0.13 in units of nanovolts/√hertz. A 10 kΩ resistor, for example, would have approximately 13 nanovolts/√hertz at room temperature.
RMS noise voltage

(A) A resistor at nonzero temperature with internal thermal noise;
(B) Its Thévenin equivalent circuit: a noiseless resistor in series with a noise voltage source;
(C) Its Norton equivalent circuit: a noiseless resistance in parallel with a noise current source.
The square root of the mean square voltage yields the root mean square (RMS) voltage observed over the bandwidth :
A resistor with thermal noise can be represented by its Thévenin equivalent circuit (Figure 4B) consisting of a noiseless resistor in series with a gaussian noise voltage source with the above RMS voltage.
Around room temperature, 3 kΩ provides almost one microvolt of RMS noise over 20 kHz (the human hearing range) and 60 Ω·Hz for corresponds to almost one nanovolt of RMS noise.
RMS noise current
A resistor with thermal noise can also be converted into its Norton equivalent circuit (Figure 4C) consisting of a noise-free resistor in parallel with a gaussian noise current source with the following RMS current:
Thermal noise on capacitors
Ideal capacitors, as lossless devices, do not have thermal noise. However, the combination of a resistor and a capacitor (an RC circuit, a common low-pass filter) has what is called kTC noise. The noise bandwidth of an RC circuit is When this is substituted into the thermal noise equation, the result has an unusually simple form as the value of the resistance (R) drops out of the equation. This is because higher R decreases the bandwidth as much as it increases the noise.
The mean-square and RMS noise voltage generated in such a filter are:
The noise charge is the capacitance times the voltage:
This charge noise is the origin of the term "kTC noise". Although independent of the resistor's value, 100% of the kTC noise arises in the resistor. Therefore, it would incorrect to double-count both a resistor's thermal noise and its associated kTC noise, and the temperature of the resistor alone should be used, even if the resistor and the capacitor are at different temperatures. Some values are tabulated below:
| Capacitance | Charge noise | ||
|---|---|---|---|
| as coulombs | as electrons | ||
| 1 fF | 2 mV | 2 aC | 12.5 e |
| 10 fF | 640 μV | 6.4 aC | 40 e |
| 100 fF | 200 μV | 20 aC | 125 e |
| 1 pF | 64 μV | 64 aC | 400 e |
| 10 pF | 20 μV | 200 aC | 1250 e |
| 100 pF | 6.4 μV | 640 aC | 4000 e |
| 1 nF | 2 μV | 2 fC | 12500 e |
Reset noise
An extreme case is the zero bandwidth limit called the reset noise left on a capacitor by opening an ideal switch. Though an ideal switch's open resistance is infinite, the formula still applies. However, now the RMS voltage must be interpreted not as a time average, but as an average over many such reset events, since the voltage is constant when the bandwidth is zero. In this sense, the Johnson noise of an RC circuit can be seen to be inherent, an effect of the thermodynamic distribution of the number of electrons on the capacitor, even without the involvement of a resistor.
The noise is not caused by the capacitor itself, but by the thermodynamic fluctuations of the amount of charge on the capacitor. Once the capacitor is disconnected from a conducting circuit, the thermodynamic fluctuation is frozen at a random value with standard deviation as given above. The reset noise of capacitive sensors is often a limiting noise source, for example in image sensors.
Any system in thermal equilibrium has state variables with a mean energy of kT/2 per degree of freedom. Using the formula for energy on a capacitor (E = 1/2CV), mean noise energy on a capacitor can be seen to also be 1/2CkT/C = kT/2. Thermal noise on a capacitor can be derived from this relationship, without consideration of resistance.
Thermometry
The Johnson–Nyquist noise has applications in precision measurements, in which it is typically called "Johnson noise thermometry".
For example, the NIST in 2017 used the Johnson noise thermometry to measure the Boltzmann constant with uncertainty less than 3 ppm. It accomplished this by using Josephson voltage standard and a quantum Hall resistor, held at the triple-point temperature of water. The voltage is measured over a period of 100 days and integrated.
This was done in 2017, when the triple point of water's temperature was 273.16 K by definition, and the Boltzmann constant was experimentally measurable. Because the acoustic gas thermometry reached 0.2 ppm in uncertainty, and Johnson noise 2.8 ppm, this fulfilled the preconditions for a redefinition. After the 2019 redefinition, the kelvin was defined so that the Boltzmann constant is 1.380649×10 J⋅K, and the triple point of water became experimentally measurable.
Thermal noise on inductors
Inductors are the dual of capacitors. Analogous to kTC noise, a resistor with an inductor results in a noise current that is independent of resistance:
Maximum transfer of noise power
The noise generated at a resistor can transfer to the remaining circuit. The maximum power transfer happens when the Thévenin equivalent resistance of the remaining circuit matches . In this case, each of the two resistors dissipates noise in both itself and in the other resistor. Since only half of the source voltage drops across any one of these resistors, this maximum noise power transfer is:
This maximum is independent of the resistance and is called the available noise power from a resistor.
Available noise power in decibel-milliwatts
Signal power is often measured in dBm (decibels relative to 1 milliwatt). Available noise power would thus be in dBm. At room temperature (300 K), the available noise power can be easily approximated as in dBm for a bandwidth in hertz. Some example available noise power in dBm are tabulated below:
| Bandwidth | Available thermal noise power at 300 K (dBm) |
Notes |
|---|---|---|
| 1 Hz | −174 | |
| 10 Hz | −164 | |
| 100 Hz | −154 | |
| 1 kHz | −144 | |
| 10 kHz | −134 | FM channel of 2-way radio |
| 100 kHz | −124 | |
| 180 kHz | −121.45 | One LTE resource block |
| 200 kHz | −121 | GSM channel |
| 1 MHz | −114 | Bluetooth channel |
| 2 MHz | −111 | Commercial GPS channel |
| 3.84 MHz | −108 | UMTS channel |
| 6 MHz | −106 | Analog television channel |
| 20 MHz | −101 | WLAN 802.11 channel |
| 40 MHz | −98 | WLAN 802.11n 40 MHz channel |
| 80 MHz | −95 | WLAN 802.11ac 80 MHz channel |
| 160 MHz | −92 | WLAN 802.11ac 160 MHz channel |
| 1 GHz | −84 | UWB channel |
Nyquist's derivation of ideal resistor noise
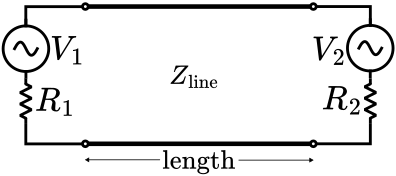
Nyquist then imagined shorting both ends of the line, thereby trapping in-flight energy on the line. Because all in-flight energy is now completely reflected (due to the now-mismatched impedance), the in-flight energy can be represented as a summation of sinusoidal standing waves. For a band of frequencies , there are modes of oscillation. Each mode provides of energy on average, of which is electric and is magnetic, so the total energy in that bandwidth on average is Each resistor contributed (half of that total energy).
But since before the shorting there were originally no reflections, the value of that total in-flight energy also equals the combined energy that was transferred from both resistors to the line during the transit time interval of . Dividing the average energy transferred from each resistor to the line by the transit time interval results in a total power of transferred over bandwidth on average from each resistor.
Nyquist's 1928 paper "Thermal Agitation of Electric Charge in Conductors" used concepts about potential energy and harmonic oscillators from the equipartition law of Boltzmann and Maxwell to explain Johnson's experimental result. Nyquist's thought experiment summed the energy contribution of each standing wave mode of oscillation on a long lossless transmission line between two equal resistors (). According to the conclusion of Figure 5, the total average power transferred over bandwidth from and absorbed by was determined to be:
Simple application of Ohm's law says the current from (the thermal voltage noise of only ) through the combined resistance is , so the power transferred from to is the square of this current multiplied by , which simplifies to:
Setting this equal to the earlier average power expression allows solving for the average of over that bandwidth:
Nyquist used similar reasoning to provide a generalized expression that applies to non-equal and complex impedances too. And while Nyquist above used according to classical theory, Nyquist concluded his paper by attempting to use a more involved expression that incorporated the Planck constant (from the new theory of quantum mechanics).
Generalized forms
The voltage noise described above is a special case for a purely resistive component for low to moderate frequencies. In general, the thermal electrical noise continues to be related to resistive response in many more generalized electrical cases, as a consequence of the fluctuation-dissipation theorem. Below a variety of generalizations are noted. All of these generalizations share a common limitation, that they only apply in cases where the electrical component under consideration is purely passive and linear.
Complex impedances
Nyquist's original paper also provided the generalized noise for components having partly reactive response, e.g., sources that contain capacitors or inductors. Such a component can be described by a frequency-dependent complex electrical impedance . The formula for the power spectral density of the series noise voltage is
The function is approximately 1, except at very high frequencies or near absolute zero (see below).
The real part of impedance, , is in general frequency dependent and so the Johnson–Nyquist noise is not white noise. The RMS noise voltage over a span of frequencies to can be found by taking the square root of integration of the power spectral density:
- .
Alternatively, a parallel noise current can be used to describe Johnson noise, its power spectral density being
where is the electrical admittance; note that
Quantum effects at high frequencies or low temperatures
With proper consideration of quantum effects (which are relevant for very high frequencies or very low temperatures near absolute zero), the multiplying factor mentioned earlier is in general given by:
At very high frequencies (), the function starts to exponentially decrease to zero. At room temperature this transition occurs in the terahertz, far beyond the capabilities of conventional electronics, and so it is valid to set for conventional electronics work.
Relation to Planck's law
Nyquist's formula is essentially the same as that derived by Planck in 1901 for electromagnetic radiation of a blackbody in one dimension—i.e., it is the one-dimensional version of Planck's law of blackbody radiation. In other words, a hot resistor will create electromagnetic waves on a transmission line just as a hot object will create electromagnetic waves in free space.
In 1946, Robert H. Dicke elaborated on the relationship, and further connected it to properties of antennas, particularly the fact that the average antenna aperture over all different directions cannot be larger than , where λ is wavelength. This comes from the different frequency dependence of 3D versus 1D Planck's law.
Multiport electrical networks
Richard Q. Twiss extended Nyquist's formulas to multi-port passive electrical networks, including non-reciprocal devices such as circulators and isolators. Thermal noise appears at every port, and can be described as random series voltage sources in series with each port. The random voltages at different ports may be correlated, and their amplitudes and correlations are fully described by a set of cross-spectral density functions relating the different noise voltages,
where the are the elements of the impedance matrix . Again, an alternative description of the noise is instead in terms of parallel current sources applied at each port. Their cross-spectral density is given by
where is the admittance matrix.
Notes
- This article is using "one-sided" (positive-only frequency) not "two-sided" frequency.
- The charge of a single electron is e− (the negative of the elementary charge). So each number to the left of e− represents the total number of electrons that make up the noise charge.
- A standing wave occurs with frequency equal to every integer multiple of . The line is sufficiently long to make the number of modes within the bandwidth very large, such that the modes will be close enough in frequency to approximate a continuous frequency spectrum.
See also
References
- John R. Barry; Edward A. Lee; David G. Messerschmitt (2004). Digital Communications. Sprinter. p. 69. ISBN 9780792375487.
- ^ Dörfel, G. (2012-08-15). "The early history of thermal noise: The long way to paradigm change". Annalen der Physik. 524 (8): 117–121. doi:10.1002/andp.201200736. ISSN 0003-3804.
- Van Der Ziel, A. (1980-01-01), "History of Noise Research", in Marton, L.; Marton, C. (eds.), Advances in Electronics and Electron Physics Volume 50, vol. 50, Academic Press, pp. 351–409, doi:10.1016/s0065-2539(08)61066-5, ISBN 978-0-12-014650-5, retrieved 2024-03-16
- Anonymous (1927). "Minutes of the Philadelphia Meeting December 28, 29, 30, 1926". Physical Review. 29 (2): 350–373. Bibcode:1927PhRv...29..350.. doi:10.1103/PhysRev.29.350.
- ^ Johnson, J. (1928). "Thermal Agitation of Electricity in Conductors". Physical Review. 32 (97): 97–109. Bibcode:1928PhRv...32...97J. doi:10.1103/physrev.32.97.
- ^ Nyquist, H. (1928). "Thermal Agitation of Electric Charge in Conductors". Physical Review. 32 (110): 110–113. Bibcode:1928PhRv...32..110N. doi:10.1103/physrev.32.110.
- ^ Lundberg, Kent H. "Noise Sources in Bulk CMOS" (PDF). p. 10.
- Sarpeshkar, R.; Delbruck, T.; Mead, C. A. (November 1993). "White noise in MOS transistors and resistors" (PDF). IEEE Circuits and Devices Magazine. 9 (6): 23–29. doi:10.1109/101.261888. S2CID 11974773.
- White, D R; Galleano, R; Actis, A; Brixy, H; Groot, M De; Dubbeldam, J; Reesink, A L; Edler, F; Sakurai, H; Shepard, R L; Gallop, J C (August 1996). "The status of Johnson noise thermometry". Metrologia. 33 (4): 325–335. doi:10.1088/0026-1394/33/4/6. ISSN 0026-1394.
- Qu, Jifeng; Benz, Samuel P; Coakley, Kevin; Rogalla, Horst; Tew, Weston L; White, Rod; Zhou, Kunli; Zhou, Zhenyu (2017-08-01). "An improved electronic determination of the Boltzmann constant by Johnson noise thermometry". Metrologia. 54 (4): 549–558. doi:10.1088/1681-7575/aa781e. ISSN 0026-1394. PMC 5621608. PMID 28970638.
- "Noise, Temperature, and the New SI". NIST (Press release). 2016-11-15.
- "NIST 'Noise Thermometry' Yields Accurate New Measurements of Boltzmann Constant". NIST (Press release). 2017-06-29.
- Fischer, J; Fellmuth, B; Gaiser, C; Zandt, T; Pitre, L; Sparasci, F; Plimmer, M D; de Podesta, M; Underwood, R; Sutton, G; Machin, G; Gavioso, R M; Ripa, D Madonna; Steur, P P M; Qu, J (2018). "The Boltzmann project". Metrologia. 55 (2): 10.1088/1681–7575/aaa790. doi:10.1088/1681-7575/aaa790. ISSN 0026-1394. PMC 6508687. PMID 31080297.
- ^ Pierce, J. R. (1956). "Physical Sources of Noise". Proceedings of the IRE. 44 (5): 601–608. doi:10.1109/JRPROC.1956.275123. S2CID 51667159.
- Vizmuller, Peter (1995), RF Design Guide, Artech House, ISBN 0-89006-754-6
- Tomasi, Wayne (1994). Electronic Communication. Prentice Hall PTR. ISBN 9780132200622.
- Callen, Herbert B.; Welton, Theodore A. (1951-07-01). "Irreversibility and Generalized Noise". Physical Review. 83 (1): 34–40. doi:10.1103/PhysRev.83.34.
- Urick, V. J.; Williams, Keith J.; McKinney, Jason D. (2015-01-30). Fundamentals of Microwave Photonics. John Wiley & Sons. p. 63. ISBN 9781119029786.
- Dicke, R. H. (1946-07-01). "The Measurement of Thermal Radiation at Microwave Frequencies". Review of Scientific Instruments. 17 (7): 268–275. Bibcode:1946RScI...17..268D. doi:10.1063/1.1770483. PMID 20991753. S2CID 26658623.
- Twiss, R. Q. (1955). "Nyquist's and Thevenin's Theorems Generalized for Nonreciprocal Linear Networks". Journal of Applied Physics. 26 (5): 599–602. Bibcode:1955JAP....26..599T. doi:10.1063/1.1722048.
![]() This article incorporates public domain material from Federal Standard 1037C. General Services Administration. Archived from the original on 2022-01-22. (in support of MIL-STD-188).
This article incorporates public domain material from Federal Standard 1037C. General Services Administration. Archived from the original on 2022-01-22. (in support of MIL-STD-188).
External links
- Amplifier noise in RF systems Archived 2008-07-04 at the Wayback Machine
- Thermal noise (undergraduate) with detailed math
 with
with  is
is  , then its
, then its  is
is  in general, where
in general, where  is the
is the  , a
, a  passes only the shaded area of height
passes only the shaded area of height  at
at  and
and 
 is the
is the  in units of nanovolts/√hertz. A 10 kΩ resistor, for example, would have approximately 13 nanovolts/√hertz at room temperature.
in units of nanovolts/√hertz. A 10 kΩ resistor, for example, would have approximately 13 nanovolts/√hertz at room temperature.

 corresponds to almost one nanovolt of RMS noise.
corresponds to almost one nanovolt of RMS noise.

 When this is substituted into the thermal noise equation, the result has an unusually simple form as the value of the
When this is substituted into the thermal noise equation, the result has an unusually simple form as the value of the 

 is the
is the 



 results in a noise current that is independent of resistance:
results in a noise current that is independent of resistance:

 can transfer to the remaining circuit. The
can transfer to the remaining circuit. The  of the remaining circuit
of the remaining circuit 
 in dBm. At room temperature (300 K), the available noise power can be easily approximated as
in dBm. At room temperature (300 K), the available noise power can be easily approximated as  in dBm for a bandwidth in hertz. Some example available noise power in dBm are tabulated below:
in dBm for a bandwidth in hertz. Some example available noise power in dBm are tabulated below:

 . Each resistor's noise
. Each resistor's noise  . All impedances are identical, so both signals are absorbed by the opposite resistor instead of being
. All impedances are identical, so both signals are absorbed by the opposite resistor instead of being 

 is electric and
is electric and  Each resistor contributed
Each resistor contributed  (half of that total energy).
(half of that total energy). . Dividing the average energy transferred from each resistor to the line by the transit time interval results in a total power of
. Dividing the average energy transferred from each resistor to the line by the transit time interval results in a total power of  transferred over bandwidth
transferred over bandwidth  ). According to the conclusion of Figure 5, the total average power transferred over bandwidth
). According to the conclusion of Figure 5, the total average power transferred over bandwidth  and absorbed by
and absorbed by  was determined to be:
was determined to be:

 (the thermal voltage noise of only
(the thermal voltage noise of only  , so the power transferred from
, so the power transferred from 
 equal to the earlier average power expression
equal to the earlier average power expression  allows solving for the average of
allows solving for the average of  over that bandwidth:
over that bandwidth:

 (from the new theory of
(from the new theory of  . The formula for the
. The formula for the 
 is approximately 1, except at very high frequencies or near absolute zero (see below).
is approximately 1, except at very high frequencies or near absolute zero (see below).
 , is in general frequency dependent and so the Johnson–Nyquist noise is not white noise. The RMS noise voltage over a span of frequencies
, is in general frequency dependent and so the Johnson–Nyquist noise is not white noise. The RMS noise voltage over a span of frequencies  to
to  can be found by taking the square root of integration of the power spectral density:
can be found by taking the square root of integration of the power spectral density:
 .
.
 is the
is the 

 ), the function
), the function  for conventional electronics work.
for conventional electronics work.
 , where λ is wavelength. This comes from the different frequency dependence of 3D versus 1D Planck's law.
, where λ is wavelength. This comes from the different frequency dependence of 3D versus 1D Planck's law.

 are the elements of the
are the elements of the  .
Again, an alternative description of the noise is instead in terms of parallel current sources applied at each port. Their cross-spectral density is given by
.
Again, an alternative description of the noise is instead in terms of parallel current sources applied at each port. Their cross-spectral density is given by

 is the
is the  . The line is sufficiently long to make the number of modes within the bandwidth very large, such that the modes will be close enough in frequency to approximate a continuous frequency spectrum.
. The line is sufficiently long to make the number of modes within the bandwidth very large, such that the modes will be close enough in frequency to approximate a continuous frequency spectrum.