| Gyroelongated pyramid | |
|---|---|
 Example pentagonal form Example pentagonal form | |
| Faces | 3n triangles 1 n-gon |
| Edges | 5n |
| Vertices | 2n + 1 |
| Symmetry group | Cnv, , (*nn) |
| Rotation group | Cn, , (nn) |
| Properties | convex |
In geometry, the gyroelongated pyramids (also called augmented antiprisms) are an infinite set of polyhedra, constructed by adjoining an n-gonal pyramid to an n-gonal antiprism.
There are two gyroelongated pyramids that are Johnson solids made from regular triangles and square, and pentagons. A triangular and hexagonal form can be constructed with coplanar faces. Others can be constructed allowing for isosceles triangles.
Forms
| Image | Name | Faces |
|---|---|---|
 |
Gyroelongated triangular pyramid (Coplanar faces) |
9+1 triangles |
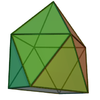 |
Gyroelongated square pyramid (J10) | 12 triangles, 1 squares |
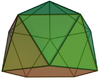 |
Gyroelongated pentagonal pyramid (J11) | 15 triangles, 1 pentagon |
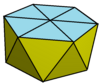 |
Gyroelongated hexagonal pyramid (Coplanar faces) |
18 triangles, 1 hexagon |
See also
References
- Norman W. Johnson, "Convex Solids with Regular Faces", Canadian Journal of Mathematics, 18, 1966, pages 169–200. Contains the original enumeration of the 92 solids and the conjecture that there are no others.
- Victor A. Zalgaller (1969). Convex Polyhedra with Regular Faces. Consultants Bureau. No ISBN. The first proof that there are only 92 Johnson solids.
| Convex polyhedra | |||||
|---|---|---|---|---|---|
| Platonic solids (regular) | |||||
| Archimedean solids (semiregular or uniform) | |||||
| Catalan solids (duals of Archimedean) |
| ||||
| Dihedral regular | |||||
| Dihedral uniform |
| ||||
| Dihedral others | |||||
| Degenerate polyhedra are in italics. | |||||
This polyhedron-related article is a stub. You can help Misplaced Pages by expanding it. |