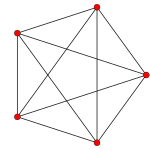
 5-cell |
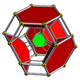
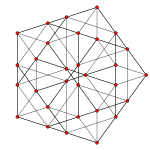 Cantellated 5-cell |
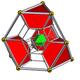
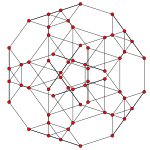 Cantitruncated 5-cell |
| Orthogonal projections in A4 Coxeter plane | ||
|---|---|---|
In four-dimensional geometry, a cantellated 5-cell is a convex uniform 4-polytope, being a cantellation (a 2nd order truncation, up to edge-planing) of the regular 5-cell.
Cantellated 5-cell
| Cantellated 5-cell | ||
|---|---|---|
 Schlegel diagram with octahedral cells shown | ||
| Type | Uniform 4-polytope | |
| Schläfli symbol | t0,2{3,3,3} rr{3,3,3} | |
| Coxeter diagram | ||
| Cells | 20 | 5 5 10 |
| Faces | 80 | 50{3} 30{4} |
| Edges | 90 | |
| Vertices | 30 | |
| Vertex figure |  Square wedge | |
| Symmetry group | A4, , order 120 | |
| Properties | convex, isogonal | |
| Uniform index | 3 4 5 | |

The cantellated 5-cell or small rhombated pentachoron is a uniform 4-polytope. It has 30 vertices, 90 edges, 80 faces, and 20 cells. The cells are 5 cuboctahedra, 5 octahedra, and 10 triangular prisms. Each vertex is surrounded by 2 cuboctahedra, 2 triangular prisms, and 1 octahedron; the vertex figure is a nonuniform triangular prism.
Alternate names
- Cantellated pentachoron
- Cantellated 4-simplex
- (small) prismatodispentachoron
- Rectified dispentachoron
- Small rhombated pentachoron (Acronym: Srip) (Jonathan Bowers)
Configuration
Seen in a configuration matrix, all incidence counts between elements are shown. The diagonal f-vector numbers are derived through the Wythoff construction, dividing the full group order of a subgroup order by removing one mirror at a time.
| Element | fk | f0 | f1 | f2 | f3 | ||||||
|---|---|---|---|---|---|---|---|---|---|---|---|
| f0 | 30 | 2 | 4 | 1 | 4 | 2 | 2 | 2 | 2 | 1 | |
| f1 | 2 | 30 | * | 1 | 2 | 0 | 0 | 2 | 1 | 0 | |
| 2 | * | 60 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | ||
| f2 | 3 | 3 | 0 | 10 | * | * | * | 2 | 0 | 0 | |
| 4 | 2 | 2 | * | 30 | * | * | 1 | 1 | 0 | ||
| 3 | 0 | 3 | * | * | 20 | * | 1 | 0 | 1 | ||
| 3 | 0 | 3 | * | * | * | 20 | 0 | 1 | 1 | ||
| f3 | 12 | 12 | 12 | 4 | 6 | 4 | 0 | 5 | * | * | |
| 6 | 3 | 6 | 0 | 3 | 0 | 2 | * | 10 | * | ||
| 6 | 0 | 12 | 0 | 0 | 4 | 4 | * | * | 5 | ||
Images
| Ak Coxeter plane |
A4 | A3 | A2 |
|---|---|---|---|
| Graph | 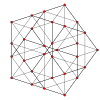
|

|
|
| Dihedral symmetry |
 Wireframe |
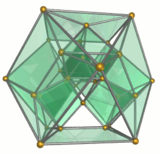 Ten triangular prisms colored green |
 Five octahedra colored blue |
Coordinates
The Cartesian coordinates of the vertices of the origin-centered cantellated 5-cell having edge length 2 are:
| Coordinates | |
|---|---|
|
|
|
The vertices of the cantellated 5-cell can be most simply positioned in 5-space as permutations of:
- (0,0,1,1,2)
This construction is from the positive orthant facet of the cantellated 5-orthoplex.
Related polytopes
The convex hull of two cantellated 5-cells in opposite positions is a nonuniform polychoron composed of 100 cells: three kinds of 70 octahedra (10 rectified tetrahedra, 20 triangular antiprisms, 40 triangular antipodiums), 30 tetrahedra (as tetragonal disphenoids), and 60 vertices. Its vertex figure is a shape topologically equivalent to a cube with a triangular prism attached to one of its square faces.
Cantitruncated 5-cell
| Cantitruncated 5-cell | ||
|---|---|---|
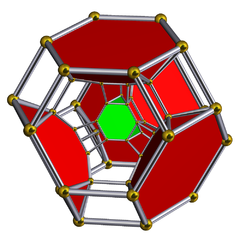 Schlegel diagram with Truncated tetrahedral cells shown | ||
| Type | Uniform 4-polytope | |
| Schläfli symbol | t0,1,2{3,3,3} tr{3,3,3} | |
| Coxeter diagram | ||
| Cells | 20 | 5 10 5 |
| Faces | 80 | 20{3} 30{4} 30{6} |
| Edges | 120 | |
| Vertices | 60 | |
| Vertex figure | 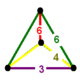 sphenoid | |
| Symmetry group | A4, , order 120 | |
| Properties | convex, isogonal | |
| Uniform index | 6 7 8 | |

The cantitruncated 5-cell or great rhombated pentachoron is a uniform 4-polytope. It is composed of 60 vertices, 120 edges, 80 faces, and 20 cells. The cells are: 5 truncated octahedra, 10 triangular prisms, and 5 truncated tetrahedra. Each vertex is surrounded by 2 truncated octahedra, one triangular prism, and one truncated tetrahedron.
Configuration
Seen in a configuration matrix, all incidence counts between elements are shown. The diagonal f-vector numbers are derived through the Wythoff construction, dividing the full group order of a subgroup order by removing one mirror at a time.
| Element | fk | f0 | f1 | f2 | f3 | |||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| f0 | 60 | 1 | 1 | 2 | 1 | 2 | 2 | 1 | 2 | 1 | 1 | |
| f1 | 2 | 30 | * | * | 1 | 2 | 0 | 0 | 2 | 1 | 0 | |
| 2 | * | 30 | * | 1 | 0 | 2 | 0 | 2 | 0 | 1 | ||
| 2 | * | * | 60 | 0 | 1 | 1 | 1 | 1 | 1 | 1 | ||
| f2 | 6 | 3 | 3 | 0 | 10 | * | * | * | 2 | 0 | 0 | |
| 4 | 2 | 0 | 2 | * | 30 | * | * | 1 | 1 | 0 | ||
| 6 | 0 | 3 | 3 | * | * | 20 | * | 1 | 0 | 1 | ||
| 3 | 0 | 0 | 3 | * | * | * | 20 | 0 | 1 | 1 | ||
| f3 | 24 | 12 | 12 | 12 | 4 | 6 | 4 | 0 | 5 | * | * | |
| 6 | 3 | 0 | 6 | 0 | 3 | 0 | 2 | * | 10 | * | ||
| 12 | 0 | 6 | 12 | 0 | 0 | 4 | 4 | * | * | 5 | ||
Alternative names
- Cantitruncated pentachoron
- Cantitruncated 4-simplex
- Great prismatodispentachoron
- Truncated dispentachoron
- Great rhombated pentachoron (Acronym: grip) (Jonathan Bowers)
Images
| Ak Coxeter plane |
A4 | A3 | A2 |
|---|---|---|---|
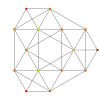
| Graph | 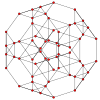
|
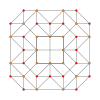
|

|
| Dihedral symmetry |
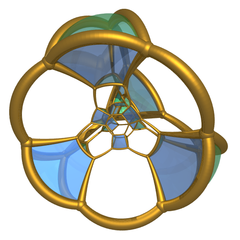 Stereographic projection with its 10 triangular prisms. |
Cartesian coordinates
The Cartesian coordinates of an origin-centered cantitruncated 5-cell having edge length 2 are:
| Coordinates | |
|---|---|
|
|
|
These vertices can be more simply constructed on a hyperplane in 5-space, as the permutations of:
- (0,0,1,2,3)
This construction is from the positive orthant facet of the cantitruncated 5-orthoplex.
Related polytopes
A double symmetry construction can be made by placing truncated tetrahedra on the truncated octahedra, resulting in a nonuniform polychoron with 10 truncated tetrahedra, 20 hexagonal prisms (as ditrigonal trapezoprisms), two kinds of 80 triangular prisms (20 with D3h symmetry and 60 C2v-symmetric wedges), and 30 tetrahedra (as tetragonal disphenoids). Its vertex figure is topologically equivalent to the octahedron.
Related 4-polytopes
These polytopes are art of a set of 9 Uniform 4-polytopes constructed from the Coxeter group.
| Name | 5-cell | truncated 5-cell | rectified 5-cell | cantellated 5-cell | bitruncated 5-cell | cantitruncated 5-cell | runcinated 5-cell | runcitruncated 5-cell | omnitruncated 5-cell |
|---|---|---|---|---|---|---|---|---|---|
| Schläfli symbol |
{3,3,3} 3r{3,3,3} |
t{3,3,3} 3t{3,3,3} |
r{3,3,3} 2r{3,3,3} |
rr{3,3,3} r2r{3,3,3} |
2t{3,3,3} | tr{3,3,3} t2r{3,3,3} |
t0,3{3,3,3} | t0,1,3{3,3,3} t0,2,3{3,3,3} |
t0,1,2,3{3,3,3} |
| Coxeter diagram |
|||||||||
| Schlegel diagram |
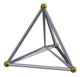
|

|

|
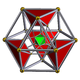
|

|
|

|
|

|
| A4 Coxeter plane Graph |

|

|

|

|

|

|

|

|

|
| A3 Coxeter plane Graph |

|

|

|

|

|

|
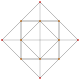
|
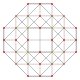
|
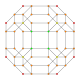
|
| A2 Coxeter plane Graph |

|
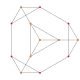
|

|

|

|

|

|
|

|
References
- Klitzing, Richard. "o3x4x3o - deca".
- Klitzing, Richard. "x3x4x3o - grip".
- H.S.M. Coxeter:
- H.S.M. Coxeter, Regular Polytopes, 3rd Edition, Dover New York, 1973
- Kaleidoscopes: Selected Writings of H.S.M. Coxeter, edited by F. Arthur Sherk, Peter McMullen, Anthony C. Thompson, Asia Ivic Weiss, Wiley-Interscience Publication, 1995, ISBN 978-0-471-01003-6
- (Paper 22) H.S.M. Coxeter, Regular and Semi Regular Polytopes I,
- (Paper 23) H.S.M. Coxeter, Regular and Semi-Regular Polytopes II,
- (Paper 24) H.S.M. Coxeter, Regular and Semi-Regular Polytopes III,
- Norman Johnson Uniform Polytopes, Manuscript (1991)
- N.W. Johnson: The Theory of Uniform Polytopes and Honeycombs, Ph.D. (1966)
- 1. Convex uniform polychora based on the pentachoron - Model 4, 7, George Olshevsky.
- Klitzing, Richard. "4D uniform polytopes (polychora)". x3o3x3o - srip, x3x3x3o - grip
| Fundamental convex regular and uniform polytopes in dimensions 2–10 | ||||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Family | An | Bn | I2(p) / Dn | E6 / E7 / E8 / F4 / G2 | Hn | |||||||
| Regular polygon | Triangle | Square | p-gon | Hexagon | Pentagon | |||||||
| Uniform polyhedron | Tetrahedron | Octahedron • Cube | Demicube | Dodecahedron • Icosahedron | ||||||||
| Uniform polychoron | Pentachoron | 16-cell • Tesseract | Demitesseract | 24-cell | 120-cell • 600-cell | |||||||
| Uniform 5-polytope | 5-simplex | 5-orthoplex • 5-cube | 5-demicube | |||||||||
| Uniform 6-polytope | 6-simplex | 6-orthoplex • 6-cube | 6-demicube | 122 • 221 | ||||||||
| Uniform 7-polytope | 7-simplex | 7-orthoplex • 7-cube | 7-demicube | 132 • 231 • 321 | ||||||||
| Uniform 8-polytope | 8-simplex | 8-orthoplex • 8-cube | 8-demicube | 142 • 241 • 421 | ||||||||
| Uniform 9-polytope | 9-simplex | 9-orthoplex • 9-cube | 9-demicube | |||||||||
| Uniform 10-polytope | 10-simplex | 10-orthoplex • 10-cube | 10-demicube | |||||||||
| Uniform n-polytope | n-simplex | n-orthoplex • n-cube | n-demicube | 1k2 • 2k1 • k21 | n-pentagonal polytope | |||||||
| Topics: Polytope families • Regular polytope • List of regular polytopes and compounds | ||||||||||||








































