This is an old revision of this page, as edited by UtherSRG (talk | contribs) at 23:30, 2 January 2004 (fix link). The present address (URL) is a permanent link to this revision, which may differ significantly from the current revision.
Revision as of 23:30, 2 January 2004 by UtherSRG (talk | contribs) (fix link)(diff) ← Previous revision | Latest revision (diff) | Newer revision → (diff)
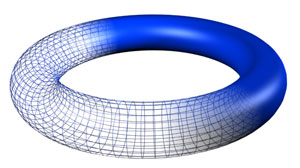
In geometry, a torus is a solid of revolution generated by revolving a circle about an axis coplanar with the circle. The sphere is a special case of the torus obtained when the axis of rotation is a diameter of the circle. If the axis of rotation does not intersect the circle, the torus has a hole in the middle and resembles a ring doughnut, a hula hoop or an inflated tyre (U.S. tire). The other case, when the axis of rotation is a chord of the circle, produces a sort of squashed sphere resembling a round cushion. Torus was the Latin word for a cushion of this shape.
According to the broadest definition, the generator of a torus need not be a circle but could also be an ellipse or any other conic section.
File:Torus1.gifpicture of torus
with one homology class
In topology torus means the product of a number of circles, the surface of a doughnut shape being the product of two. (In proper mathematical usage, a solid as described above would be spoken of as generated from a disk, i.e., a filled-in circle.) An algebraic torus in the theory of algebraic groups is so-called not directly for topological reasons, but more on account of the analogy with the way actual tori play a role in the theory of compact Lie groups (which begins from the idea of maximal torus).
In nuclear physics a torus is a large fusion reactor which is very roughly the shape of an elliptical torus. Examples are JET in the UK, JT-60 in Japan, TFTR in the USA and the proposed ITER.