| Revision as of 07:26, 2 April 2009 editHeadbomb (talk | contribs)Edit filter managers, Autopatrolled, Extended confirmed users, Page movers, File movers, New page reviewers, Pending changes reviewers, Rollbackers, Template editors454,789 edits title fields← Previous edit | Revision as of 07:37, 2 April 2009 edit undoHeadbomb (talk | contribs)Edit filter managers, Autopatrolled, Extended confirmed users, Page movers, File movers, New page reviewers, Pending changes reviewers, Rollbackers, Template editors454,789 edits ref tweakNext edit → | ||
| Line 654: | Line 654: | ||
| |author=B.I. Halperin, D.R. Nelson | |author=B.I. Halperin, D.R. Nelson | ||
| |title=Dislocation-mediated melting in two dimensions | |title=Dislocation-mediated melting in two dimensions | ||
| |journal=Phys. Rev. | |journal=Phys. Rev. B | ||
| |volume= |
|volume=19 |page=2457 | ||
| |year=1979 | |year=1979 | ||
| |doi= | |doi= | ||
| Line 662: | Line 662: | ||
| |author=A.I. Zippelius | |author=A.I. Zippelius | ||
| |title=Dynamics of two-dimensional melting | |title=Dynamics of two-dimensional melting | ||
| |journal=Phys. Rev. | |journal=Phys. Rev. B | ||
| |volume= |
|volume=22 |page=2514 | ||
| |year=1980 | |year=1980 | ||
| |doi= | |doi= | ||
| Line 692: | Line 692: | ||
| |author=P.J. Steinhardt, D.R. Nelson, M. Ronhetti | |author=P.J. Steinhardt, D.R. Nelson, M. Ronhetti | ||
| |title=Bond-orientational order in liquids and glasses | |title=Bond-orientational order in liquids and glasses | ||
| |journal=Phys. Rev. | |journal=Phys. Rev. B | ||
| |volume= |
|volume=28 |page=784 | ||
| |year=1983 | |year=1983 | ||
| |doi= | |doi= | ||
| Line 708: | Line 708: | ||
| |author=D.R. Nelson | |author=D.R. Nelson | ||
| |title=Order, frustration, and defects in liquids and glasses | |title=Order, frustration, and defects in liquids and glasses | ||
| |journal=Phys. Rev. | |journal=Phys. Rev. B | ||
| |volume= |
|volume=28 |page=5515 | ||
| |year=1983 | |year=1983 | ||
| |doi= | |doi= | ||
| Line 728: | Line 728: | ||
| |author=F.H. Stillinger, T.A. Weber | |author=F.H. Stillinger, T.A. Weber | ||
| |title=Hidden structure in liquids | |title=Hidden structure in liquids | ||
| |journal=Phys. Rev. | |journal=Phys. Rev. A | ||
| |volume= |
|volume=25 |page=978 | ||
| |year=1982 | |year=1982 | ||
| |doi= | |doi= | ||
| Line 880: | Line 880: | ||
| |year=1939 | |year=1939 | ||
| |doi=10.1063/1.1750497 | |doi=10.1063/1.1750497 | ||
| }}</ref><ref> | |||
| {{cite journal | |||
| |author=M. Born | |||
| |title=On the stability of crystal lattices I | |||
| |journal=Proc. Camb. Phil. Soc. | |||
| |volume=36 |page=160 | |||
| |year=1940 | |||
| |doi= | |||
| }}</ref><ref> | |||
| {{cite journal | |||
| |author=M. Born, R. Furth | |||
| |title=The stability of crystal lattices III | |||
| |journal=Proc. Camb. Phil. Soc. | |||
| |volume=36 |page=454 | |||
| |year=1940 | |||
| |doi= | |||
| }}</ref><ref> | |||
| {{cite journal | |||
| |author=R.D. Misra | |||
| |title=On the stability of crystal lattices II | |||
| |journal=Proc. Camb. Phil. Soc. | |||
| |volume=36 |page=173 | |||
| |year=1941 | |||
| |doi= | |||
| }}</ref><ref> | |||
| {{cite journal | |||
| |author=R. Furth | |||
| |title=The stability of crystal lattices | |||
| |journal=Proc. Camb. Phil. Soc. | |||
| |volume=37 |page=34 | |||
| |year=1941 | |||
| |doi= | |||
| }}</ref><ref> | |||
| {{cite journal | |||
| |author=M. Born | |||
| ⚫ | |title=On the stability of crystal lattices IX. Covariant theory of lattice deformations and the stability of some hexagonal lattices | ||
| |journal=Proc. Camb. Phil. Soc. | |||
| |volume=38 |page=82 | |||
| |year=1942 | |||
| |doi= | |||
| }}</ref><ref> | |||
| {{cite journal | |||
| |author=M. Born, R.D. Misra | |||
| |title=On the stability of crystal lattices IV | |||
| |journal=Proc. Camb. Phil. Soc. | |||
| |volume=36 |page=466 | |||
| |year=1943 | |||
| |doi= | |||
| }}</ref><ref> | |||
| {{cite journal | |||
| |author=M. Born, M. Bradburn | |||
| |title=The thermodynamics of crystal lattices | |||
| |journal=Proc. Camb. Phil. Soc. | |||
| |volume=39 |page=104 | |||
| |year=1943 | |||
| |doi= | |||
| }}</ref><ref> | |||
| {{cite journal | |||
| |author=M. Bradburn | |||
| |title=The thermodynamics of crystal lattices | |||
| |journal=Proc. Camb. Phil. Soc. | |||
| |volume=39 |page=113 | |||
| |year=1943 | |||
| |doi= | |||
| }}</ref><ref> | |||
| {{cite journal | |||
| |author=M. Born | |||
| |title=The thermodynamics of crystal lattices | |||
| |journal=Proc. Camb. Phil. Soc. | |||
| |volume=39 |page=100 | |||
| |year=1944 | |||
| |doi= | |||
| }}</ref><ref> | |||
| {{cite journal | |||
| |author=M.M. Gow | |||
| |title=The thermodynamics of crystal lattices | |||
| |journal=Proc. Camb. Phil. Soc. | |||
| |volume=40 |page=151 | |||
| |year=1944 | |||
| |doi= | |||
| }}</ref> | }}</ref> | ||
| <ref>Born, M., On the stability of crystal lattices I, Proc. Camb. Phil. Soc., Vol. 36, p. 160 (1940)</ref> | |||
| <ref>Born, M., Furth, R., The stability of crystal lattices III, Proc. Camb. Phil. Soc., Vol. 36, p. 454 (1940)</ref> | |||
| <ref>Misra, R.D., On the stability of crystal lattices II, Proc. Camb. Phil. Soc., Vol. 36, p. 173 (1941)</ref> | |||
| <ref>Furth, R., The stability of crystal lattices, Proc. Camb. Phil. Soc., Vol. 37, p. 34 (1941)</ref> | |||
| ⚫ | |||
| <ref>Born, M. and Misra, R.D., On the stability of crystal lattices IV, Proc. Camb. Phil. Soc., Vol. 36, p. 466 (1943)</ref> | |||
| <ref>Born, M., Bradburn, M., The thermodynamics of crystal lattices, Proc. Camb. Phil. Soc., Vol. 39, p. 104 (1943)</ref> | |||
| <ref>Bradburn, M. The thermodynamics of crystal lattices, Proc. Camb. Phil. Soc., Vol. 39, p. 113 (1943)</ref> | |||
| <ref>Born, M., The thermodynamics of crystal lattices, Proc. Camb. Phil. Soc., Vol. 39, p. 100 (1944)</ref> | |||
| <ref>Gow, M.M., The thermodynamics of crystal lattices, Proc. Camb. Phil. Soc., Vol. 40, p. 151 (1944)</ref> | |||
| The formation of an ] ] which is rigid and therefore resistant to macroscopic flow is dependent on the formation of a stable ] of ]. As pointed out by Kauzmann and Eyring however, in their interpretation of the viscous flow of large ]ic molecules, such bonds can become ruptured upon application of external forces exceeding the elastic ], and do not immediately recombine. Microscopic flow for forces greater than those at the yield point will depend upon the relative rates of breaking -- and of recombination of the broken bonds -- and therefore upon the mean lifetime of such bonds. As the mean lifetime becomes greater, viscous forces predominate until permanent bonds are formed at which time ] sets in. (The macroscopic mechanical properties of the resulting solid will depend upon the individual strength of such bonds and the bond density.)<ref> | The formation of an ] ] which is rigid and therefore resistant to macroscopic flow is dependent on the formation of a stable ] of ]. As pointed out by Kauzmann and Eyring however, in their interpretation of the viscous flow of large ]ic molecules, such bonds can become ruptured upon application of external forces exceeding the elastic ], and do not immediately recombine. Microscopic flow for forces greater than those at the yield point will depend upon the relative rates of breaking -- and of recombination of the broken bonds -- and therefore upon the mean lifetime of such bonds. As the mean lifetime becomes greater, viscous forces predominate until permanent bonds are formed at which time ] sets in. (The macroscopic mechanical properties of the resulting solid will depend upon the individual strength of such bonds and the bond density.)<ref> | ||
| Line 929: | Line 999: | ||
| {{cite book | {{cite book | ||
| |author=G.W. Scherer | |author=G.W. Scherer | ||
| |title= |
|title=Relaxation in Glass and Composites | ||
| |publisher=Krieger | |||
| |year=1992 | |year=1992 | ||
| |ibsn=0894646435 | |||
| }}</ref> | }}</ref> | ||
| Line 1,149: | Line 1,221: | ||
| |author=D. Levesque ''et al''. | |author=D. Levesque ''et al''. | ||
| |title=Computer "Experiments" on Classical Fluids. IV. Transport Properties and Time-Correlation Functions of the Lennard-Jones Liquid near Its Triple Point | |title=Computer "Experiments" on Classical Fluids. IV. Transport Properties and Time-Correlation Functions of the Lennard-Jones Liquid near Its Triple Point | ||
| |journal=Phys. Rev. | |journal=Phys. Rev. A | ||
| |volume= |
|volume=7 |page=1690 | ||
| |year=1973 | |year=1973 | ||
| |doi= | |doi= | ||
| Line 1,365: | Line 1,437: | ||
| |author=R.C. Zeller, R.O. Pohl | |author=R.C. Zeller, R.O. Pohl | ||
| |title=Thermal Conductivity and Specific Heat of Non-crystalline Solids | |title=Thermal Conductivity and Specific Heat of Non-crystalline Solids | ||
| |journal=Phys. Rev. | |journal=Phys. Rev. B | ||
| |volume= |
|volume=4 |page=2029 | ||
| |year=1971 | |year=1971 | ||
| |doi= | |doi= | ||
| Line 1,383: | Line 1,455: | ||
| |author=M.P. Zaitlin, M.C. Anderson | |author=M.P. Zaitlin, M.C. Anderson | ||
| |title=Phonon thermal transport in noncrystalline materials | |title=Phonon thermal transport in noncrystalline materials | ||
| |journal=Phys. Rev. | |journal=Phys. Rev. B | ||
| |volume= |
|volume=12 |page=4475 | ||
| |year=1975 | |year=1975 | ||
| |doi= | |doi= | ||
| Line 1,391: | Line 1,463: | ||
| |author=M.P. Zaitlin, L.M. Scherr, M.C. Anderson | |author=M.P. Zaitlin, L.M. Scherr, M.C. Anderson | ||
| |title=Boundary scattering of phonons in noncrystalline materials | |title=Boundary scattering of phonons in noncrystalline materials | ||
| |journal=Phys. Rev. | |journal=Phys. Rev. B | ||
| |volume= |
|volume=12 |page=4487 | ||
| |year=1975 | |year=1975 | ||
| |doi= | |doi= | ||
| Line 1,504: | Line 1,576: | ||
| |author=M. Jonson, S.M. Girvin | |author=M. Jonson, S.M. Girvin | ||
| |title= | |title= | ||
| |journal=Phys. Rev. | |journal=Phys. Rev. B | ||
| |volume= |
|volume=22 |page=3283 | ||
| |year=1980 | |year=1980 | ||
| |doi= | |doi= | ||
| Line 1,516: | Line 1,588: | ||
| |author=D. Turnbull | |author=D. Turnbull | ||
| |title= | |title= | ||
| |journal=J. Phys. | |journal=J. Phys. C | ||
| |volume= |
|volume=35 |page=1 | ||
| |year=1974 | |year=1974 | ||
| |doi= | |doi= | ||
| Line 1,534: | Line 1,606: | ||
| |author=R. Wang, D. Merz | |author=R. Wang, D. Merz | ||
| |title=Polymorphic bonding and thermal stability of elemental noncrystalline solids | |title=Polymorphic bonding and thermal stability of elemental noncrystalline solids | ||
| |journal=Phys. Stat. Sol. |
|journal=Phys. Stat. Sol. A | ||
| |volume= |
|volume=39 |page=697 | ||
| |year=1977 | |year=1977 | ||
| |doi= | |doi= | ||
Revision as of 07:37, 2 April 2009
The physics of glass is the science of the glassy or amorphous state of matter as seen from an atomic or molecular point of view. The purpose of this article is to provide a description of glass: a solid in which no significant crystallization has occurred. Thus there is no long-range ordering or extended formation of any Bravais lattice.
Introduction
Generally speaking, the atomic or molecular structure of glass exists in a metastable state with respect to its crystalline form. This essentially reflects their formation from a non-equilibrium supercooled liquid state. Fundamental principles of Gibbs free energy minimization dictate this thermodynamic driving force towards crystallinity, long-range symmetry and thermodynamic equilibrium.
Since the early theoretical and experimental investigations on polymorphism and the various states of aggregation which can be assumed by a given substance, the vitreous state of matter has been recognized as having the mechanical response of both solid and liquid, depending on the time and length scale under consideration. This explains the age-old quandary of whether to label it as solid or liquid.
The purpose of this article is to describe the physical nature of glass, with emphasis on both liquid-like and solid-like behavior. The duality of its physical nature makes clear the debate and confusion over whether to call it solid or liquid. The solution to this riddle lies in the realization and acceptance of the concept that it possesses the physical properties of both solid and liquid on different scales of length and time. Thus, rather than discrediting any claims of either in exclusion of the other, it is rather the intent to fully credit the majority of all claims of either with the inclusion of both.
From the standpoint of particle physics, the kinetic theory of gases only holds for independent particles that have no interactions with other particles. It is a rough approximation, as most gases exist as diatoms (e.g. H2, N2, Cl2, etc.) or even larger clusters (e.g. S2, S4, S5, S6, S8). At standard temperature and pressure (STP), only the noble gases are monatomic. But for most gases, it is a first approximation. And more importantly, it is instrumental in formulating a description of the gaseous state of matter using macroscopic thermodynamic variables such as pressure, P, volume, V, and temperature, T.
Consider the equation of state (or ideal gas law):
In 1873, van der Waals introduced parameters to allow for both attraction and repulsion (or "effective molecular volume"). While the equation is definitely superior to the ideal gas law and does predict the formation of a liquid phase, the agreement with experimental data is limited for conditions where the liquid forms. (See better approximations here).
Consider next what might constitute an effective equation of state for an elastic crystalline solid. For example, in order to effectively describe the heat capacity of the solid, one would need a complete spectrum of vibrational frequencies for a perfect monatomic crystalline solid. In his 1911 article entitled A Relationship between Elastic Behavior and Specific Heat in (Simple) Solids, Einstein proposed a first approximation based on a Planck's Law distribution of independent harmonic oscillators. It should be pointed out that such equations would not even be valid for nearest neighbor particle-particle interactions. Improvements represent a serious challenge mathematically. This area remains as one the great unsolved problems in the most comprehensive texts in the fields of physical chemistry, solid state physics, and thus condensed matter physics.
Thus, as materials scientists, we have no equations of state for either liquids or solids (much less glasses). But we do have basic particle physics which still apply to the spatial scales under consideration for atoms and molecules. We also have a reasonable understanding of the normal modes of vibration in an elastic crystalline solid ( see Einstein solid and Debye model ) from the longest wavelength (or fundamental frequency of the body) to the highest Debye frequency (that of a single particle). Furthermore, we have simple equations to describe the relationship of these normal modes to the mechanisms of thermal phonon wave propagation as represented by the superposition of elastic waves -- both longitudinal (acoustic) and transverse (optical) waves of atomic displacement.
It is from this standpoint that we approach a microscopic description of the viscosity and viscoelastic behavior of simple fluids, and their relationship to the mechanisms of vitrification and the glass transition, with the ultimate goal of shedding some light on the continuing debate over the true nature of the vitreous state of matter.
Structure
Domains and Defects
The effects of structure on transport phenomena in liquids were considered by Eyring et al. in their attempt to develop a partition function for the liquid state. Utilizing the "hole" or "free volume" theory of non-associated liquids, Eyring attempted to explain viscosity, plasticity and diffusion in liquids as the formation and filling of molecularly sized holes. In a manner analogous to that proposed by Einstein for a crystalline solid in his theory of specific heats, each atom is regarded by Eyring as moving independently in the field of all other atoms, which are regarded as being at rest in thelr mean positions, thus surrounding the atom considered.
In calculating the partition function of liquid mercury, Eyring treats the atoms as hard spheres and ascribes to each sphere a certain "free volume", which is the volume traced out due to thermal displacements of the center of gravity of a molecule from its equilibrium position. He subsequently accounts for the low entropy of fusion (latent heat divided by temperature of fusion) of the alkali metals by assuming that the frequency of the atomic vibrations increases on melting, owing to the volume increase. It has been pointed out by Frenkel, however, that the average oscillation frequency will be a decreasing function of the volume.
A more satisfactory approximation has been used by Lennard-Jones, who, making use of known interatomic forces (as opposed to hard spheres) calculates the field in which any one atom will move if the others arc at rest in their mean positions.
Criticism of the statistical approach of both Eyring and Lennard-Jones appeared in the form of a note (by Mott and Gurney) emphasizing the absence in the theories of any place for the concept of a varying degree of order. Additional criticism was aimed at the lack of consideration of the possibility of any continuous transition from the solid to the liquid phase. It was pointed out that Bernal had attempted to show on geometrical grounds that no continuous transition from the crystalline to the amorphous or liquid state is possible without passing through the polycrystalline state, in which regular regions are separated by regions of misfit.
It was therefore proposed that a liquid is nothing more than the limiting state of a polycrystalline solid in which the individual grains or domains are so small that one cannot draw any sharp distinction between the surfaces or misfit. This suggestion was made in the interest of improvement over the interpretation made by Eyring of the entropy of fusion for the liquid state. Thus, the energy of the aggregate is assumed to be proportional to the total area of the surfaces of misfit, and the entropy approximated accordingly.
Subsequent papers by Lennard-Jones et al. on cooperative phenomena emphasized the order-disorder transition of melting, utilizing the statistical approach of the Bragg-Williams model. The mathematical model used involves the assumption that the "force" tending to produce order at a given point is uniquely determined by the average state of order throughout the crystal. As pointed out by Bethe, this "force" will actually depend on the configuration of the atoms in the immediate neighborhood of the point under consideration. The B-W model was appropriately modified, and has been utilized extensively in the treatment of atomic ratios in alloys and critical points in liquids and solids. It should be noted, however, that the potential for such statistical treatments in the elucidation of the physical mechanisms responsible for such phenomena is highly limited.
Mott's notion of ordered domains being present in the liquid state was formalized by Frenkel in his theory of heterophase density fluctuations. According to this theory, fluctuations in particle density with configurations resembling that of the crystalline state will exist in a liquid at temperatures above the melting point. An equilibrium distribution of such fluctuations will be in a constant state of growth and dissolution, and will not, therefore, require the simultaneous collision of the total number of particles in each domain.
The crystallite theory of liquids and its consequences on melting and viscous flow was considered in a series of papers by Ookawa, who emphasized that the specific heat is one of the macroscopic properties that truly reflects the mode of thermal motion of the primary particles in different states of aggregation. He interprets specific heat measurements as indicating that the thermal agitation of the constituent elements of a liquid is nearly vibrational (solid-like) at low temperature, but almost non-vibrational as high temperature. He goes on to show that the domain size will be a decreasing function of temperature. The non-vibrational or translational degrees of freedom at higher temperatures are the result of a degeneration of some relatively low frequency vibrational degrees of freedom, caused by the relaxation of the rigid coupling of the domains at their interfaces. Large domains are shown to move virtually independently with a corresponding independent translational mode.
Smaller domains, alternatively, are seen to be strongly coupled with a resulting cooperative motion. In systems with a finite compressibility the degree of correlations between the displacements of neighboring crystallites will decrease with the distance of separation. Such correlated displacements are shown to be necessary in the consideration of the dynamic properties of the liquid under a sufficiently large external force. A constant of proportionality exists (the viscosity) between the rate of shear strain and the applied force in normal Newtonian liquids, and consideration of correlations are unnecessary. But under sufficient external force, the proportionality is lost as a certain time period is necessary for the cooperative relative shear movement of many domains, with non-Newtonian effects becoming manifest.
In either case, translational motion at the inter-crystalline boundaries is accompanied by the relative shear movement of the crystallites. The motion of the boundary, which is assumed to be non-directional in the natural state, becomes predominant in one direction in a shear stressed state, resulting in a macroscopic flow of the system.
This interpretation of boundary motion is similar to that of Mott, who suggests that the fluidity of a liquid is due only to the presence of a large number of such mobile crystal defects. Mott's interpretation of melting or loss of rigidity of a polycrystalline mass is therefore based on the point at which boundaries between individual grains can move freely through the aggregate. Such a suggestion is not dissimilar from that of Frenkel that the viscous flow of a liquid and its crystallization both take place by the same mechanism of diffusion.
Dislocations and melting

The notion of inter-crystalline or grain boundaries being composed of proper arrays of dislocations led to the next logical step in the sequence of events leading to an understanding of flow mechanisms in liquids as well as solids. MacKenzie and Mott suggested the possibility that melting results from a sudden proliferation or dislocations. This led Shockley to establish a relationship between the mobility of a dislocation and the fluidity of a liquid in quantitative manner, by assuming a value for the effective dislocation concentration in the liquid state, together with certain assumptions regarding dislocation width. Sears subsequently refuted Andrade's explanation of viscosity, claiming that viscosity anomalies in supercooled liquids result from the growth of solid-like nuclei via a screw dislocation mechanism. Cahn elaborated on this topic by postulating that in sufficiently supercooled systems a liquid-solid interface can advance normal to itself in the absence of any such heterogeneities.
Kurosawa's study suggested that the contribution of lattice defects is more important than that of lattice vibrations in the melting of ionic crystals, indicating that the Coulombic interaction between defects can cause a type of phase transition to occur. Kuhlmannn-Wilsdorf, emphasizing that the state of a dislocation core is more liquid than solid-like, suggested the pairing of dislocations whose Burgers vectors were of equal magnitude but opposite sign. This dislocation dipole has the important characteristic that the long-range strain fields of the two members of the dipole cancel each other. Her resulting theory of meiting90a is based on the contention that the free energy of glide dislocation cores becomes negative, resulting in a loss of all resistance to shear forces. It was further shown that a crystal containing a sufficiently high concentration of dislocations will have a pair correlation function resembling that of a liquid.
The theoretical study of dynamic events occurring on extremely short time scales (such as that of atomic vibrations or defect translations) was advanced considerably through the computer simulation technique of Molecular Dynamics. The first such study of melting (by Cotterill and coworkers) included the observation of the spontaneous generation of dislocation pairs. The theory of dislocation-mediated melting was then reviewed. These studies were extended into the stable liquid where it was concluded that the heterogeeneous distribution of "free volume" in a Lennard-Jones liquid could not be explained on the basis of vacancies, and that the inevitable dislocations must be in a state of continuous motion. (A similar approach has since been utilized to study the glass transition.)
The heterogeneous free volume description (domains vs. defects) was considered also by Turnbull and Cohen in order to describe molecular transport in liquids and glasses. Utilizing the free volume model developed by Doolittle to describe viscous flow in Newtonian liquids, a relationship between the diffusion coefficient and the free volume is proposed based upon the concept that statistical redistribution of the free volume occasionally opens up voids large enough for diffusive displacement of a molecule which is typically confined within a molecular cage. Glass formation occurs when the rate of cooling exceeds the time necessary for such diffusion to occur, thus limiting the molecular motion. This approach has been generalized by Cohen and Grest in the development of a formal treatment of relaxation processes in liquids and glasses. Other defect-diffusion models have also been developed which are based on the proposed existence of one mechanism for relaxation.
Thus, at any molecular site, relaxation can only occur when a defect diffuses to the site. This approach was used by Phillips et al. to describe volume-controlled relaxations in viscoelastic media. A similar model proposed by Gilman for viscous flow via dislocation motion in liquids was utilized by Cotterill et al. to develop a unified theory of melting, crystallization and glass formation. Such defect theories of liquid viscosity and glass formation may be criticized with respect to the necessity of postulating an ordered structure at the atomic or molecular level in order to define a Burgers vector. It should additionally be pointed out that the presence of any mechanism of plastic flow in the glassy state, such as the "dislocations" identified by Levengood and Vong on fracture surfaces, is seriously questionable in all materials except glassy metals which have been referred to by Gilman as "ideal" glasses.
Two-dimensional computer simulation techniques have been utilized by Kosterlitz and Thouless to generalize the dislocation model to include magnetic and superfluid transitions utilizing a renormalization group treatment of the many dipole situation and relating this to 2D melting. In their model, the topological order of a solid phase is destroyed by the dissociation of dislocation pairs.
Hexatic fluid phase
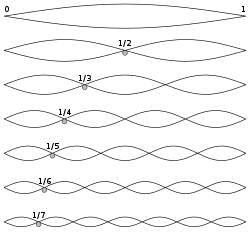
The previously described approach was extended by Halperin and Nelson, who showed that a liquid crystal-like phase, referred to as a "hexatic fluid phase", should intervene between the crystalline and isotropic fluid states. The first-order melting transition is then replaced by two second-order transitions. One of the main contributions of this theory is the decomposition of the dislocation on a triangular lattice into a more primary defect: the disclination (see figure). Thus the dislocation can be viewed as consisting of five- and seven- coordinated disclinations spaced one Janice parameter apart. The dissociation of dislocation pairs is, therefore, followed by the dissociation of disclination pairs and the two second-order transitions obtain the liquid state.
- Note: Consider an elementary dislocation on a triangular lattice. The Burgers vector is the amount by which the path around the singularity fails to close. The pattern would be a closed circuit on a perfect lattice. The 6 coordinated dislocation can be broken down into individual 5 and 7 coordinated disclinations which are all separated by one lattice spacing.
This approach was extended further by Chaudhari et al. at the IBM Watson Research Center to study defect stability and bond orientational order in supercooled liquids. It was established much earlier by Frank that an icosahedral clustering of twelve atoms about a central sphere is energetically more stable than crystalline configurations for Lennard-Jones pair potentials. These studies concluded that structural fluctuations in liquids below the melting point suggest extended correlations in the orientations of local icosahedral packing units. Nelson later emphasized the role of such noncrystalline packing units in the "frustration" of the crystallization path. This frustration was thus identified as the mechanism of glass formation from metallic liquids.
Hidden structure
A different approach has been taken by Stillinger and Weber at Bell Labs, who have identified static "hidden structure" in liquids and explored the dynamics of structural transitions in liquids. Utilizing Molecular Dynamics, they have separated the study of the liquid state into two parts: the identification of mechanically stable packings of molecules via potential minima, followed by taking account of vibrational motion (generally anharmonic) about those mechanically stable points. All configurations are "quenched" by a steepest-descent construction into a nearby potential minimum. The systems exhibit a "defect softening" phenomenon, or mean attraction between defects, which influences the spectrum of normal mode vibrational frequencies at the local potential minima for liquids that solidify into body centered cubic (BBC) crystals. Attempts to reconstitute the equilibrium pair correlations functions by thermally broadening the quenched versions, using Einstein or Debye approximations, were clear failures. Evidently, the true broadening phenomena in such systems entails substantial anharmonicity.
The presence of "hidden structure' in supercooled liquids has been verified by the electron microscope studies of Zarzycki and Mezard who have indicated a well defined "micellar" structure of glass which is interpreted as being the result of a superlattice of paracrystalline domains. The geometrical disorder of glass is therefore only exhibited at length scales above 100 angstroms (approximately the size of the elementary domain). Various degrees of interdomain ordering can therefore be realized.
This conceptualization of the paracrystalline nature of glass was further substantiated by the "domain microscopy" of Chen and Phillips et al., at Bell Labs who observed domains as large as 1000 angstroms in thin films of chalcogenide alloys. These observations led Phillips to interpret glass formaation as the aggregation of molecular clusters upon a reduction of the amplitude of thermal motion, yielding a nearly polycrystalline microstructure.
Dynamics
Viscosity of simple liquids
The mechanisms of atomic/molecular motion (or particle displacement) in solid materials are closely related to the mechanisms of viscous flow and solidification in liquid materials. Early attempts at correlating the change in flow behavior of many liquids with temperature were rationalized by Macleod who assumed that the viscosity is a simple function of the "free space" within the liquid, or the amount of space in which individual atoms or molecules are free to move. Thus, thermal expansion or contraction would yield a corresponding increase or decrease in the fluidity of the liquid, respectively.
Variations of this theme were necessary for consideration of substances whose molecules are known to be "associated" in the liquid state at ordinary temperatures, a condition referred to by Stewart as the cybotactic condition of molecular groupings. Thus, when various molecules combine together to form an associated molecule, they enclose within a semi-rigid system a certain amount of space which before was available as free space in which individual molecules could move. It is therefore suggested that the more rapid increase in viscosity upon cooling is due to the tendency of most substances to become associated on cooling,
Similar arguments could be used to describe the effects of pressure on viscosity, where it may be assumed that the viscosity is chiefly a function of the volume for liquids with a finite compressibility. An increasing rate of rise of viscosity with rise of pressure is therefore to be expected. In addition, if the volume is expanded by heat but reduced again by pressure, the viscosity remains the same.
The inner mechanism of structural transformations (or diffusionless transformations) in liquids is a subject which was given considerable attention by Andrade who believed that the theory of liquids should be approached not from the point of view of the kinetic theory of gases, which was constructed to deal with matter where the spaces between the molecules are large compared with the size of molecules, but from the point of view of the solid state, the density of which is not markedly different from that of the liquid state of the same substance. The validity of such an approach is clearly evidenced by the fact that the viscosity of a liquid decreases while the viscosity of a gas increases with rising temperature.
In a gas, the tangential forces between two parallel layers are produced by the transport of the individual atoms from one layer to another, with a consequent momentum transfer. Between collisions, the molecules are supposed to move freely over a distance large compared to the molecular size. In liquids, on the other hand, there is no mean free path at all, the motion of a molecule being always in an intense field of intermolecular force.

Andrade emphasizes that the intermolecular forces in the solid and the liquid state cannot be very different, and cites Lindemann's theory of melting, which has been remarkably successful in yielding accurate values for the atomic vibrational frequencies of the normal modes of vibration of simple solids. Lindemann supposes that melting occurs when the amplitude of the vibrations of the atoms about their equilibrium positions becomes a fixed large fraction of the interatomic separation distance. Loss of mechanical equilibrium yields fluidity, but clearly the liquid in the neighborhood of its melting point has much in common with the solid.
Andrade therefore considers a liquid moving in plane layers parallel to one another, there being a velocity gradient in the direction normal to the layers. In contrast to Maxwell's theory of gaseous viscosity, which is based upon movement of the equilibrium positions of molecules from one layer to another, Andrade assumes that the viscosity is due to a temporary union at the periphery of molecules in adjacent layers, due to their large amplitudes of vibration. The viscosity is therefore a result of communication of momentum which is associated with a temporary state of bonding, each atom or molecule retaining its association with its original position, which will change slowly.
The essential difference between the liquid and solid state is therefore not the magnitude of the intermolecular force under which the molecule vibrates, but the amplitude of the motion. In the liquid state, this is so large that the molecules come into contact at every extreme libration, a result of which is that they are disturbed and the "position of equilibrium", which in a crystalline solid is fixed, is in a liquid slowly displaced. The assumption is, then, that there is for a liquid molecule a vibration about a slowly displaced equilibrium position, with a frequency which is the same as that of molecules in the solid state.
It is therefore assumed that when, at the maximum of the vibrational amplitude, the molecules of one layer come into contact with those of an adjacent layer, they will, in a large fraction of such collisions, enter into a temporary union. The duration of this union must not exceed the very brief time necessary for the molecules to acquire a common velocity of translation. The duration must be very small compared to the period of vibration, and does not involve anything of the kind that is ordinarily called association. This sharing of velocities between molecules in adjacent layers, and the corresponding transfer or communication of momentum, will introduce viscous forces in the manner described in Maxwell's original theory of viscosity in gases.
It is further pointed out that communication of momentum takes place only if the mutual potential energy, probably determined by the relative orientation of the approaching molecules, is favorable. The tendency to orientation, which is favorable for interchange of momentum, is disturbed by thermal agitation. A certain mutual potential energy is therefore necessary if communication of momentum is to take place in the direction normal to the line of centers; that is, if the molecules are to behave momentarily as one. This is a direct consequence of the fact that the potential energy of molecule in the field of intermolecular force must depend upon its orientation. It is then supposed that there is a local electrostatic field which tends to place the molecules in a condition favorable for communication of momentum, or to orient them. The conditions of this orientation are disturbed by thermal agitation with the corresponding effects of temperature on viscosity.
The local tendency to orientation of molecules in small groups lends the liquid (as referred to previously)a certain degree of association. As emphasized by MacLeod such an association can result in a considerable "internal pressure" within a liquid. He states that it is plausible to regard the internal pressure as being almost entirely due to those molecules which, on account of their temporary low velocities (following the Maxwell distribution of velocities) have coalesced with other molecules or are in very close association with them. The internal pressure between several such molecules would be very great compared with that between several average molecules and might correspond to the internal pressure between a group of molecules in the solid form.
The rationale utilized by Macleod is indeed reminiscent of that in the classic paper by Herzfeld and Geoppert Mayer on the elastic theory of fusion, where it is pointed out that the heat of fusion is the same order of magnitude as the elastic energy necessary to expand a crystalline lattice to the breaking point. Mechanical stability is thus dependent on a balance between the "elastic tension" (negative) which is related to the potential energy of the lattice and the 'thermal pressure" (positive) which is associated with the kinetic energy of the normal modes of vibration of the lattice. The thermal pressure (which must be included in the equation of state at temperatures above absolute zero) is determined essentially by the change in vibrational frequency with volume. Thus, localized changes in specific volume resulting from association can result in local pressure gradients internally due to thermal vibration.
Structural relaxation
The fact that a solid deforms while retaining its rigidity while a liquid yields to macroscopic viscous flow on the application of a shearing force is accepted by many as the mechanical distinction between the two. Thus, it may not be without reason to compare, with Born, et al. the melting point of solids with the temperature at which the modulus of rigidity (shear modulus) vanishes. But it is to be emphasized that the rigidity and the fluidity are not exclusive properties to each other.
The formation of an elastic network which is rigid and therefore resistant to macroscopic flow is dependent on the formation of a stable network of chemical bonds. As pointed out by Kauzmann and Eyring however, in their interpretation of the viscous flow of large polymeric molecules, such bonds can become ruptured upon application of external forces exceeding the elastic limit, and do not immediately recombine. Microscopic flow for forces greater than those at the yield point will depend upon the relative rates of breaking -- and of recombination of the broken bonds -- and therefore upon the mean lifetime of such bonds. As the mean lifetime becomes greater, viscous forces predominate until permanent bonds are formed at which time elasticity sets in. (The macroscopic mechanical properties of the resulting solid will depend upon the individual strength of such bonds and the bond density.)
Frenkel took this argument a step further by considering the dynamics of thermal motion of atoms about their static equilibrium positions in the rigid elastic network. The rigidity of crystals is in full agreement with the conception that this 'heat motion' reduces to vibrations of small amplitude about invariable equilibrium positions. This conception seems to be wholly inappropriate, however, in the case of liquids with their characteristic fluidity.
A way out of this contradiction lies in the assumption that the positions of the atoms in a liquid body are not permanent, but have a temporary (or temporal) nature. After performing a more or less larger number of oscillations about the same position during a specific time interval, t, each atom of the liquid can jump to a new position where it is surrounded, partially at least, by new neighbors. If the time t is large compared with the period of vibration of the atom, P, this displacement of position (or translation) will not affect the magnitude of the specific heat of the liquid, which will remain solid-like. If, however, at the same time, P is small compared with the time scale during which the body is acted upon by a force of constant magnitude and direction, it will yield to this force with a consequent microscopic flow.
In the contrary case, when P is large compared with the time scale of the external force, the same force will produce only an elastic deformation of the body. Thus, the characteristic fluidity of liquids can be displayed only under the action of forces which vary sufficiently slowly with respect to magnitude, and especially to direction, such that the time of their action in a given direction is large compared with the average value of t, the mean lifetime of an atom in its equilibrium position. Under such conditions the rigidity of liquids, (their capability of elastic resistance to a shearing stress) connected with the finite lifetimes of temporary equilibrium positions, is masked by their apparent `fluidity.
Frenkel identified the mean lifetime of an atom in its equilibrium position with the relaxation time originally described in Maxwell's kinetic theory of gases. The structural rearrangement necessary for the termination of the atom's residence in this position has resulted in an extension of the terminology to "structural relaxation" which has received much attention in certain academic circles in the study of non-equilibrium phenomenon. In the simplest case of a monatomic liquid, the structural relaxation must reduce to a change of the degree of local order in the packing morphology of the particles, in the sense of a more compact arrangement of higher density when the liquid is compressed or a more open distribution of lower density when it is expanded.
This change of the degree of local order must in general lag with respect to the variation of the volume (or the pressure), since it is connected with a rearrangement of the particles or a redistribution of their mutual orientations -- or with processes requiring a certain activation energy, and proceeding accordingly with a finite velocity. This is the origin of viscous relaxation due to microscopic structural transformations.
Viscoelastic behavior
This description of the rigidity or elasticity of liquids is obviously limited to very short time scales. The identification may be substantiated by a consideration of the influence of an externally applied sinusoidal or harmonically varying force. If the period of vibration of the force is small (or conversely if the vibrational frequency is high) compared with the time of structural relaxation, then such experiments would probe the fundamental forces responsible for the elastic vibrations of the body. Thus, even most simple liquids will exhibit some elastic response at frequencies or shear rates exceeding 5 x 10 Hz. Alternatively, if the vibrational period of the force is large (low frequency) compared with the relaxation time, then the vibrational motion of the body will partially degenerate into translational motion, and the resulting displacement will be evidenced by microscopic viscous flow.
Materials which respond to mechanical disturbances by both viscous (or plastic/irreversible) and elastic (or reversible) behavior under distinct ranges of deformation (and rate deformation, or frequency) are called viscoelastic. Thus, when a mechanical force is applied suddenly to a fluid, the fluid responds elastically at first, just as if it were a solid body. Whether the rigidity or the fluidity predominates in a material under given conditions is therefore determined by the time scale of the experiment relative to the characteristic time of structural relaxation of the material.
Zwanzig and Mountain calculated the high-frequency elastic moduli of simple fluids by considering the pressure and internal energy of the fluid. They concluded that the initial response to a sudden disturbance can be characterized by two quantities:
- The high-frequency limit of the shear modulus G (or modulus of rigidity)
- The high-frequency limit of the bulk modulus K (or modulus of compression).
The connection between a viscous and an elastic response is made by considering the stress for a disturbance varying periodically in time with a frequency, υ. For consideration of shear flow, it is supposed that the shear viscosity q(υ) is a function of the frequency, and is related to the relaxation time t, which is characteristic of the transition from elastic to viscous response. High-frequency disturbances are identified as those relating to elastic behavior, while low-frequency behavior is identified as ordinary viscous flow. Thus the frequency-dependent viscosity coefficient q(υ) is capable of describing both viscous and elastic phenomena, and can be related to the frequency-dependent elastic moduli, K(υ) and G(υ).
It is interesting to note that at zero frequency, the shear modulus vanishes and the response can be described by the bulk modulus (inverse compressibility) alone. Fleury refers to this condition as the thermodynamic limit (υ --> 0), in considering the high-frequency dynamics of simple liquids and solids near their melting points. The remarkable conclusions of his inelastic light scattering studies near the melting point is that there is no discernible difference between the liquid and solid vibrational spectra at sufficiently high frequencies. This means that on the short time and length scales probed by these experiments, melting causes no discontinuous change in the microscopic dynamics of the substance. The lower the frequency, the larger the discontinuity between liquid and solid behavior -- so that in the thermodynamic limit (zero frequency) the transition is first order.
Vitrification
Since the early theoretical and experimental investigations on polymorphism and the various states of aggregation which can be assumed by a given substance, the vitreous state of a substance has been recognized as having the mechanical response of both solid and liquid, depending on the time and spatial scale under consideration. The atomic arrangement of network-forming oxides in the vitreous or glassy state was described by Zachariasen as exhibiting the disorder (or short-range order) of its liquid precursor and therefore defying description through any crystalline hypotheses. The random network description of the internal structure was extended by Warren with the topological characteristics of the Zachariasen network subsequently being investigated by Cooper.
A multitude of substances including polymers, network forming oxides, and metallic alloys have now been found to exhibit glass-forming characteristics, and it is of interest to discuss the criterion which separates the characteristics of glass forming ability from the typically observed nucleation and growth of a crystalline phase.
Zachariasen suggested that the ultimate condition for the formation of a glass is that the substance can form extended three-dimensional networks lacking periodicity with an energy content comparable with that of the corresponding crystal network. Viscous flow characteristics of liquids near the glass transition temperature were reviewed by Jones and interpreted in terms of configurational changes at the molecular level.
Kauzmann subsequently pointed out that the metastable nature of the glassy state is associated with the freezing-in of certain internal degrees of freedom in the liquid, while Davies and Jones proceeded to formalize a treatment of the thermodynamics and kinetics of the irreversible approach to equilibrium in glasses in terms of such macroscopic thermodynamic variables as the volume, enthalpy and entropy. Such treatments led to subsequent controversy over the use of irreversible thermodynamics in the treatment of glass formation, and the order (first or second) of the macroscopic phase transition. Such questions remain highly controversial in scientific circles today.
The difficulties involved in developing a fundamental understanding of the nature of the glass transition itself are similar to those involved in the understanding of any rate-controlled phenomena, such as chemical reactions. A distinction should be drawn between an empirical description of the kinetics of the reaction, and a physical understanding of the reaction itself. The latter involves a picture of its mechanism, i.e., the path of the reaction.
This point is emphasized by Weyl and Marboe in their dynamic interpretation of vitrification. Thus, the kinetic treatment of glass formation by Uhlmann which is based upon the kinetic equations for phase changes developed by Avrami should be contrasted, for example, with the mechanical description of vitrification given by Bartenev. The latter emphasizes the localized nature of the distribution of microscopic stress and strain upon vitrification.
It is important to note that the dynamics of any rate-controlled phenomena can only be understood if one considers the nature of the motion of its primary constituents. Thus, thermal motion in liquids can be decomposed into elementary longitudinal waves (or acoustic phonons), while transverse waves (or shear waves) were originally described (and observed) only in the crystalline state. This is the fundamental reason why simple liquids cannot support a shearing stress, but rather yield via macroscopic flow. The inadequacies of this conclusion, however, were pointed out by Frenkel in his revision of the theory of elasticity of liquids. This revision follows directly from the continuous character of the transition from the liquid state into the solid one when this transition is not accompanied by crystallization and long-range atomic and/or molecular ordering (or self-assembly).
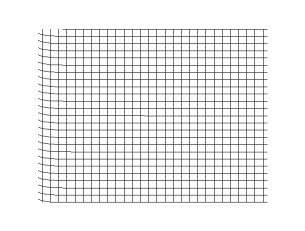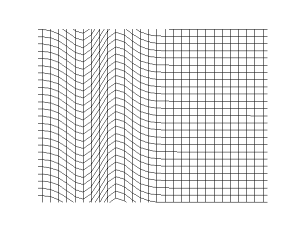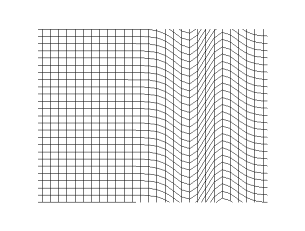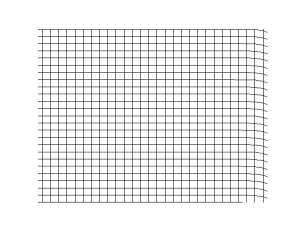
It can be concluded from Frenkel's observations on continuity that transverse vibrations can be propagated not only in crystalline bodies, but also in liquids. The fact that this conclusion is not verified experimentally in the case of ordinary liquids is due to the short time scale of relaxation compared to the period of vibrations which can be obtained with the help of modern opto-acoustic methods: lasers and ultrasonics. Under such conditions, the transverse vibrations must be strongly damped.
Experimental verification of Frenkel's conclusions have been obtained using Molecular Dynamics computer simulation studies in monatomic liquids and glasses where it has been shown that, at short wavelengths, monatomic liquids can support a propagating shear wave, i.e. a collective excitation which is analogous to the transverse phonons found in solids. The onset of this viscoelastic behavior is linked to the fact that as the wave number increases the rigidity of the liquid becomes an important factor.
Mechanisms of attenuation of high-frequency shear modes and longitudinal waves were considered by Mason et al. at Bell Labs with viscous liquids, polymers and glasses. The subsequent work in the Physics Department of the Catholic University of America led to an entirely new interpretation of the glass transition in viscous liquids in terms of a spectrum of structural relaxation phenomena occurring over a wide range of spatial and temporal scales. Experimentally, the use of Dynamic Light Scattering experiments (or Photon Correlation Spectroscopy) makes possible the study of molecular processes from time intervals as short as 10 sec. This is equivalent to extending the available frequency range to 10 Hz or greater.
Thus we see the intimate correlation between transverse acoustic phonons (or shear waves) and the onset of rigidity or vitrification. The frequency dependence of this phenomenon becomes apparent when one considers the increasing wavelength over which such rigidity can be observed. The relationship between these transverse waves and the mechanism of vitrification has been described by Chen, et a1. who proposed that the onset of correlations between such phonons results in an orientational ordering or "freezing" of local shear stresses in liquids, yielding the glass transition.
The representation of thermal motion in liquids as a superposition of elastic sound waves was first introduced by Brillouin. Atomic motion in condensed matter can therefore be represented by a Fourier series whose physical interpretation consists of a superposition of supersonic longitudinal and transverse atomic displacement waves( or density fluctuations) with varying directions and wavelengths.
With respect to sound wave propagation, the speed of longitudinal or compression waves will be limited by the bulk modulus of compressibility. The square root of the ratio of the bulk modulus K to the density p will be equal to the velocity of propagation of longitudinal phonons. In the case of transverse vibrations or shear waves, for which the density remains constant, the speed of such waves will be limited by the shear modulus or rigidity.
The square root of the ratio of the shear modulus G to the density will be equal to the velocity of transverse phonons. Thus, the wave velocities will be given by:
where the constant of proportionality ρ in both cases is the particle density or reciprocal specific volume.
The velocities of longitudinal acoustic phonons in condensed matter are directly responsible for the thermal conductivity which levels out temperature differentials between compressed and expanded volume elements. So Kittel proposed that the behavior of glasses is interpreted in terms of an approximately constant mean free path for lattice phonons, and that the value of the mean free path is of the order of magnitude of the scale of disorder in the structure at the atomic or molecular level.
Klemens subsequently emphasized that heat transport in dielectric solids occurs through elastic vibrations of the lattice, and that this transport is limited by elastic scattering of acoustic phonons by lattice defects. These predictions were confirmed by the experiments of Chang and Jones on commercial glasses and glass ceramics, where mean free paths were limited by "internal boundary scattering" to length scales of 10 cm to 10 cm.
Klemens further identified this phonon mean free path with the effective relaxation length for processes without directional correlation. Thus, if Vg is the group velocity of a phonon wave packet, then the relaxation length is defined as:
where t is the characteristic relaxation time. Now, since longitudinal waves have a much greater group or "phase velocity" than that of transverse waves, V(long.) is much greater than V (trans.), the relaxation length or mean free path of longitudinal phonons will be much greater. Thus, thermal conductivity will be largely determined by the speed of longitudinal phonons.
The dependence of wave velocity on wavelength or frequency, referred to as dispersion, was also addressed by Klemens, who suggested that low-frequency phonons of long wavelength will be limited in relaxation length by elastic Rayleigh scattering which will be proportional to the fourth power of the frequency. For higher frequencies, the power of the frequency will decrease until at highest frequencies scattering is almost frequency independent. According to Klemens, such higher frequency phonons will be scattered by "defect clusters".
Similar arguments were subsequently generalized to many substances in the glassy state by Zeller and Pohl and confirmed by the technique of Brillouin scattering (thermal generation of phonons with detection by light scattering) by Love.
Subsequent experiments by Zaitlin et al. were contrasted with those theories interpreting thermal resistance as being due to scattering of sound waves by lattice defects in crystals. In this context, diffuse scattering of phonons is attributed rather to the general "roughness" of the amorphous structure which is said not to contain such well defined lattice defects.
The thermal phonon mean free paths or relaxation lengths of a number of glass formers have now been plotted versus the glass transition temperature T, by Reynolds who has shown a linear relationship between the two. Thus, Reynolds has suggested a new criterion for glass formation based on the value of the phonon mean free path.
The influence of thermal phonons, and their interaction with electronic structure, is a topic which was appropriately introduced by Sir Nevill Mott in his classic paper on the resistance of liquid metals. He refers to Lindemann's theory of melting and suggests that the drop in conductivity in going from the crystalline to the liquid state is due to the increased scattering of conduction electrons as a result of the increased amplitude of atomic vibration. Such theories of localization have been applied to transport in metallic glasses, where the mean free path of the electrons is very small, on the order of the interatomic spacing.
The formation of a noncrystalline form of a gold-silicon alloy by Klement, et a1.in 1960 by the method of splat quenching from the melt led to further considerations of the influence of electronic structure on glass forming ability based on the properties of the metallic bond.Reviews on the subject of metallic glasses are provided here.
Johnson and Girvin argue that the mobility of localized electrons is enhanced by the presence of dynamic phonon modes. One claim against such a model is that if chemical bonds are important, the nearly free electron models should not be applicable. However, if the model includes the buildup of a charge distribution between all pairs of atoms just like a chemical bond (e.g., silicon, when a band is just filled with electrons) then it should apply to solids.
Thus, if the electrical conductivity is low, the mean free path of the electrons is very short. The electrons will only be sensitive to the short-range order in the glass since they do not get a chance to scatter from atoms spaced at large distances. Since the short-range order is similar in glasses and crystals, the electronic energies should be similar in these two states. For alloys with lower resistivity and longer electronic mean free paths, the electrons could begin to sense that there is disorder in the glass, and this would raise their energies and destabilize the glass with respect to crystallization. Thus, the glass formation tendencies of certain alloys may therefore be due in part to the fact that the electron mean free paths are very short, so that only the short-range order is ever important for the energy of the electrons.
Turnbull has argued that glass formation in metallic systems is related to the "softness" of the interaction potential between unlike atoms. Chen and Park, emphasizing the strong similarities between the local structure of the glass and the corresponding crystal, suggest that chemical bonding helps to stabilize the amorphous structure.
Wang and Merz have suggested that the electronic structure yields its influence on glass formation through the directional properties of bonds. Non-crystallinity is thus favored in elements with a large number of polymorphic forms and a high degree of bonding anisotropy. Crystallization becomes more unlikely as bonding anisotropy is increased from isotropic metallic to anisotropic metallic to covalent bonding, thus suggesting a relationship between the group number in the periodic table and the glass forming ability in elemental solids.
See also
- Sol-gel
- Waves
- Phonons
- Acoustics
- Vitreous
- Nucleation
- Viscoelasticity
- Glass transition
- Colloidal crystal
- Ceramic engineering
- Ceramics processing
- Brillouin scattering
- Transparent materials
- Dynamic light scattering
- Crystallographic defect
- Glass transition temperature
- Phase transformations in solids
- Viscosity of amorphous materials
References
- J.H. Gibbs (1960). "Chapter 7". In J.D. MacKenzie (ed.). Modern Aspects of the Vitreous State. Butterworth. OCLC 1690554.
-
C. Moynihan; et al. (1976). The Glass Transition and the Nature of the Glassy State. New York Academy of Sciences. ISBN 0890720533.
{{cite book}}: Explicit use of et al. in:|author=(help); Unknown parameter|editors=ignored (|editor=suggested) (help) - J. Wong, A.C. Angell (1976). Glass Structure by Spectroscopy. Marcel Dekker. ISBN 0824764684.
- P. Atkins (1994). Physical Chemistry (5th ed.). W.H. Freeman. ISBN 0198557302.
- J.D. van der Waals (1988) . On the Continuity of the Gaseous and Liquid States (Ph.D. Thesis). Dover. ISBN 0486495930.
- A. Einstein (1911). "Beziehung zwischen dem elastischen Verhalten und der Spezifischen Wärme mit einatomigem Molekül". Ann. Der Physik. 34 (1): 170. doi:10.1002/andp.200590028.
- A. Einstein (1911). "Elementare Betrachtungen über die thermische Molekularbewegung in festen Körpern". Ann. Der Physik. 35 (9): 679. doi:10.1002/andp.19113400903.
-
A. Einstein (1907). "Plancksche Theorie der Strahlung und die Theorie der Spezifischen Wärme". Ann. Der Physik. 22: 180. doi:10.1002/andp.200590013.
with correction A. Einstein (1907). "Berichtigung zu meiner Arbeit: Die Plancksche Theorie der Strahlung etc". Ann. Der Physik. 22: 800. doi:10.1002/andp.19073270415. - P. Debye (1912). "Zur Theorie der spezifischen Wärme". Ann. Der Physik. 39: 789.
-
M. Born, L. von Karman (1912). Phys. Zeitschr. 13: 297.
{{cite journal}}: Missing or empty|title=(help) -
M. Born, L. von Karman (1913). Phys. Zeitschr. 14: 15.
{{cite journal}}: Missing or empty|title=(help) - M. Blackman (1935). "Contributions to the Theory of the Specific Heat of Crystals. I. Lattice Theory and Continuum Theory". Proc. Roy. Soc. A. 148: 365. doi:10.1098/rspa.1935.0024.
- M. Blackman (1935). "Contributions to the Theory of the Specific Heat of Crystals. II. On the Vibrational Spectrum of Cubical Lattices and Its Application to the Specific Heat of Crystals". Proc. Roy. Soc. A. 148: 384. doi:10.1098/rspa.1935.0025.
- M. Blackman (1935). "Contributions to the Theory of Specific Heat. III. On the Existence of Pseudo-T Regions in the Specific Heat Curve of a Crystal". Proc. Roy. Soc. A. 149: 117. doi:10.1098/rspa.1935.0051.
- M. Blackman (1935). "Contributions to the Theory of Specific Heat. IV. On the Calculation of the Specific Heat of Crystals from Elastic Data". Proc. Roy. Soc. A. 149: 126. doi:10.1098/rspa.1935.0052.
- M. Blackman (1937). "On the Vibrational Spectrum of a Three Dimensional Lattice". Proc. Roy. Soc. A. 159: 416. doi:10.1098/rspa.1937.0081.
- M. Blackman (1937). "Some Properties of the Vibrational Spectrum of a Lattice". Math. Proc. Camb. Phil. Soc. 33: 94. doi:10.1017/S0305004100016819.
- ^ L. Brillouin (1922). "Diffusion de la lumière et des rayons X par un corps transparent homogène; influence de l'agitation thermique". Ann. Phys. 17: 88.
-
N.F. Mott, H. Jones (1958). Theory of the Properties of Metals and Alloys. Dover. ISBN 0486604560.
{{cite book}}: Check|isbn=value: checksum (help); Unknown parameter|orig=ignored (help) - J.C. Slater (1970) . Introduction to Chemical Physics. Dover. ISBN 0486625621.
- F. Seitz (1987) . Modern Theory of Solids. Dover. ISBN 0486654826.
- C. Kittel (1986). Introduction to Solid State Physics (6th ed.). John Wiley & Sons. ISBN 0471874744.
- H. Eyring, J. Hirschfelder (1937). "The Theory of the Liquid State". J. Phys. Chem. 41: 249. doi:10.1021/j150380a007.
- H. Eyring (1936). "Viscosity, Plasticity, and Diffusion as Examples of Absolute Reaction Rates". J. Chem. Phys. 4: 283. doi:10.1063/1.1749836.
- J.F. Kincaid, H. Eyring (1937). "A Partition Function for Liquid Mercury". J. Chem. Phys. 5: 587. doi:10.1063/1.1750078.
- ^
J. Hirschfelder, D. Stevenson, H. Eyring (1937). "A Theory of Liquid Structure". J. Chem. Phys. 5: 896. doi:10.1063/1.1749960.
{{cite journal}}: CS1 maint: multiple names: authors list (link) - J.E. Lennard-Jones, A.F. Devonshire (1937). "Critical Phenomena in Gases I. Vapour Pressures and Boiling Points". Proc. Roy. Soc. London A. 163: 53. doi:10.1098/rspa.1937.0210.
- J.E. Lennard-Jones, A.F. Devonshire (1938). "Critical Phenomena in Gases II. Vapour Pressures and Boiling Points". Proc. Roy. Soc. London A. 165: 1. doi:10.1098/rspa.1938.0039.
- N.F. Mott, R.W. Gurney (1939). "Note on the theory of liquids". Trans. Far. Soc. 35: 364. doi:10.1039/tf9393500364.
- J.D. Bernal (1937). "An attempt at a molecular theory of liquid structure". Trans. Far. Soc. 33: 27. doi:10.1039/tf9373300027.
-
J.E. Lennard-Jones, A.F. Devonshire, (1939). "Critical and Co-Operative Phenomena III. A Theory of Melting and the Structure of Liquids". Proc. Roy. Soc. London A. 169: 317. doi:10.1098/rspa.1939.0002.
{{cite journal}}: CS1 maint: extra punctuation (link) CS1 maint: multiple names: authors list (link) - J.E. Lennard-Jones, A.F. Devonshire (1939). "Critical and Co-operative Phenomena IV. A Theory of Disorder in Solids and Liquids and the Process of Melting". Proc. Roy. Soc. London A. 170: 464. doi:10.1098/rspa.1939.0043.
- W.L. Bragg, E.J. Williams (1934). "The Effect of Thermal Agitation on Atomic Arrangement in Alloys I". Proc. Roy. Soc. London A. 145: 699. doi:10.1098/rspa.1934.0132.
- W.L. Bragg, E.J. Williams (1935). "The Effect of Thermal Agitation on Atomic Arrangement in Alloys II". Proc. Roy. Soc. London A. 151: 540. doi:10.1098/rspa.1935.0165.
- E.J. Williams (1935). "The Effect of Thermal Agitation on Atomic Arrangement in Alloys III". Proc. Roy. Soc. London A. 152: 231. doi:10.1098/rspa.1935.0188.
- H.A. Bethe (1935). "Statistical Theory of Superlattices". Proc. Roy. Soc. London A. 150: 552. doi:10.1098/rspa.1935.0122.
- J. Frenkel (1939). "A General Theory of Heterophase Fluctuations and Pretransition Phenomena". J. Chem. Phys. 7: 538. doi:10.1063/1.1750484.
- A. Ookawa (1933). "Crystallite Theory of Liquid I: On the Specific Heat". J. Phys. Soc. Jpn. 2: 108. doi:10.1143/JPSJ.2.108.
- A. Ookawa (1934). "Crystallite Theory of Liquid, II. Solid-Liquid Transformation". J. Phys. Soc. Jpn. 3: 295. doi:10.1143/JPSJ.3.295.
- ^ A. Ookawa (1949). "Crystallite Theory of Liquid. III. The Viscous Flow". J. Phys. Soc. Jpn. 4: 14. doi:10.1143/JPSJ.4.14.
- J.K. MacKenzie, N.F. Mott (1950). "A Note on the Theory of Melting". Proc. Phys. Soc. A. 65: 411. doi:10.1088/0370-1298/63/4/114.
- W. Shockley (1952) L'État Solide, Inst. Int'l. de Physique, Solvay, Brussels p.431
- G.W. Sears (1955). "Viscosity Anomaly of Supercooled Liquids". J. Chem. Phys. 23: 604. doi:10.1063/1.1742062.
- J.W. Cahn (1960). "Theory of Crystal Growth and Interface Motion in Crystalline Materials". Acta Met. 8: 554. doi:10.1016/0001-6160(60)90110-3.
- T. Kurosawa (1957). "On the Melting of Ionic Crystals". J. Phys. Soc. Jpn. 12: 338. doi:10.1143/JPSJ.12.338.
- D. Kuhlmann-Wilsdorf (1965). "Theory of Melting". Phys. Rev. 140: A1599. doi:10.1103/PhysRev.140.A1599.
- I.A. Kotze, D. Kuhlmann-Wilsdorf (1971). "Radial correlation functions of metallic melts calculated from structural models of the liquid state". Phil. Mag. 23: 1133. doi:10.1080/14786437108217401.
- R.M.J. Cotterill, L.B. Pedersen (1972). "A molecular dynamics study of the melting of a two-dimensional crystal". Solid State Comm. 10: 439. doi:10.1016/0038-1098(72)90914-3.
-
R.M.J. Cotterill; et al. (1973). "Molecular dynamics studies of melting I: Dislocation density and the pair distribution function". Phil. Mag. 27: 623. doi:10.1080/14786437308219236.
{{cite journal}}: Explicit use of et al. in:|author=(help) -
R.M.J. Cotterill; et al. (1974). "Molecular dynamics studies of melting II: Dislocation density and thermodynamic functions". Phil. Mag. 30: 229. doi:10.1080/14786439808206551.
{{cite journal}}: Explicit use of et al. in:|author=(help) -
R.M.J. Cotterill; et al. (1974). "A molecular dynamics approach to grain boundary structure and migration". Phil. Mag. 30: 265. doi:10.1080/14786439808206553.
{{cite journal}}: Explicit use of et al. in:|author=(help) -
E.J. Jensen; et al. (1975). "Order-Disorder Transition Produced by Dislocations in an FCC Lennard-Jones Solid". J. De Phys. 36: C2-49. doi:10.1051/jphyscol:1975209.
{{cite journal}}: Explicit use of et al. in:|author=(help) -
R.M.J. Cotterill; et al. (1979). T. Riste (ed.). Ordering in Strongly Fluctuating Condensed Matter Systems.
{{cite book}}: Explicit use of et al. in:|author=(help) -
R.M.J. Cotterill; et al. (1979). "Dislocation-like Structures in a Simulated Liquid". Phys. Rev. Lett. 42: 1541. doi:10.1103/PhysRevLett.42.1541.
{{cite journal}}: Explicit use of et al. in:|author=(help) - R.M.J. Cotterill, J.U. Madsen (1980). "Evidence against a crystal instability in melting". Nature. 288: 467. doi:10.1038/288467a0.
- R.M.J. Cotterill, J.U. Madsen (1980). "Crystal-Liquid Instability and Pressure-Induced Glass Transition". Physica Scripta. 22: 635. doi:10.1088/0031-8949/22/6/018.
- R.M.J. Cotterill, J.U. Madsen (1981). "The entropy difference between the Lennard-Jones crystalline and glassy states". Phys. Lett. A. 83: 219. doi:10.1016/0375-9601(81)90828-8.
- D. Turnbull, M.H. Cohen (1958). "Concerning Reconstructive Transformation and Formation of Glass". J. Chem. Phys. 29: 1049. doi:10.1063/1.1744654.
- D. Turnbull, M.H. Cohen (1959). "Molecular Transport in Liquids and Glasses". J. Chem. Phys. 31: 1164. doi:10.1063/1.1730566.
- D. Turnbull, M.H. Cohen (1961). "Free-Volume Model of the Amorphous Phase: Glass Transition". J. Chem. Phys. 34: 120. doi:10.1063/1.1731549.
- D. Turnbull, M.H. Cohen (1970). "On the Free-Volume Model of the Liquid-Glass Transition". J. Chem. Phys. 52: 3038. doi:10.1063/1.1673434.
- A.K. Doolittle (1951). "Studies in Newtonian Flow II. The Dependence of the Viscosity of Liquids on Free-Space". J. Appl. Phys. 22: 1471. doi:10.1063/1.1699894.
- M.H. Cohen, G.S. Grest (1979). "Liquid-glass transition: a free-volume approach". Phys. Rev. B. 20: 1077. doi:10.1103/PhysRevB.20.1077.
-
M.C. Phillips; et al. (1972). "Relaxation in Liquids: A Defect-Diffusion Model of Viscoelasticity". Proc. Roy. Soc. London A. 329: 193. doi:10.1098/rspa.1972.0108.
{{cite journal}}: Explicit use of et al. in:|author=(help) - J.J. Gilman (1973). "Flow via dislocations in ideal glasses". J. Appl. Phys. 44: 675. doi:10.1063/1.1662243.
-
R.M.J. Cotterill; et al. (1965). "A Unified Theory of Melting Crystallization and Glass Formation". J. De Phys. 36: C2–35. doi:10.1051/jphyscol:1975208.
{{cite journal}}: Explicit use of et al. in:|author=(help) - W.C. Levengood, T.S. Vong (1960). "Spiral Dislocations on Glass Fracture Surfaces". J. Appl. Phys. 31: 1416. doi:10.1063/1.1735855.
- J.M. Kosterlitz, D.J. Thouless (1972). "Long range order and metastability in two dimensional solids and superfluids: Application of dislocation theory". J. Phys. C. 5: L124. doi:10.1088/0022-3719/5/11/002.
- J.M. Kosterlitz, D.J. Thouless (1973). "Ordering, metastability and phase transitions in two-dimensional systems". J. Phys. C. 6: 1181. doi:10.1088/0022-3719/6/7/010.
- D.R. Nelson, J.M. Kosterlitz (1977). "Universal Jump in the Superfluid Density of Two-Dimensional Superfluids". Phys. Rev. Lett. 39: 1201. doi:10.1103/PhysRevLett.39.1201.
- B.I. Halperin, D.R. Nelson (1978). "Theory of Two-Dimensional Melting". Phys. Rev. Lett. 41: 121.
- B.I. Halperin, D.R. Nelson (1979). "Dislocation-mediated melting in two dimensions". Phys. Rev. B. 19: 2457.
- A.I. Zippelius (1980). "Dynamics of two-dimensional melting". Phys. Rev. B. 22: 2514.
-
P. Chaudhari, A. Levi, P.J. Steinhardt (1979). "Edge and Screw Dislocations in an Amorphous Solid". Phys. Rev. Lett. 43: 1517. doi:10.1103/PhysRevLett.43.1517.
{{cite journal}}: CS1 maint: multiple names: authors list (link) -
P.J. Steinhardt, D.R. Nelson, M. Ronhetti (1981). "Icosahedral Bond Orientational Order in Supercooled Liquids". Phys. Rev. Lett. 47: 1297. doi:10.1103/PhysRevLett.47.1297.
{{cite journal}}: CS1 maint: multiple names: authors list (link) -
P.J. Steinhardt, D.R. Nelson, M. Ronhetti (1983). "Bond-orientational order in liquids and glasses". Phys. Rev. B. 28: 784.
{{cite journal}}: CS1 maint: multiple names: authors list (link) - F.C. Frank (1952). "Supercooling of Liquids". Proc. Roy. Soc. London A. 215: 43.
- D.R. Nelson (1983). "Order, frustration, and defects in liquids and glasses". Phys. Rev. B. 28: 5515.
- F.H. Stillinger, T.A. Weber (1976). "Phase transitions in the Gaussian core system". J. Chem. Phys. 65: 3968. doi:10.1063/1.432891.
- F.H. Stillinger, T.A. Weber (1982). "Hidden structure in liquids". Phys. Rev. A. 25: 978.
- F.H. Stillinger, T.A. Weber (1984). "Inherent structures and distribution functions for liquids that freeze into bcc crystals". J. Chem. Phys. 81: 5089. doi:10.1063/1.447498.
- F.H. Stillinger, T.A. Weber (1984). "Point defects in bcc crystals: Structures, transition kinetics, and melting implications". J. Chem. Phys. 81: 5095. doi:10.1063/1.447499.
- F.H. Stillinger, T.A. Weber (1984). "Packing Structures and Transitions in Liquids and Solids". Science. 225: 983. doi:10.1126/science.225.4666.983. PMID 17783020.
-
J. Zarzycki, R. Mezard (1962). Phys. Chem. Glasses. 3: 163.
{{cite journal}}: Missing or empty|title=(help) -
C.H. Chen; et al. (1981). "Domain microscopy in chalcogenide alloy glass thin films". Solid State Comm. 38: 657.
{{cite journal}}: Explicit use of et al. in:|author=(help) -
J.C. Phillips (1982). Physics Today: 27.
{{cite journal}}: Missing or empty|title=(help) - ^ D.B. Macleod (1923). "On a relation between the viscosity of a liquid and its coefficient of expansion". Trans. Far. Soc. 19: 6. doi:10.1039/tf9231900006.
- ^ G.W Stewart (1930). "The Cybotactic (Molecular Group) Condition in Liquids; the Nature of the Association of Octyl Alcohol Molecules". Phys. Rev. 35: 726. doi:10.1103/PhysRev.35.726.
- ^ E.N. Andrade (1934). "Kinetic Theory of Liquids". Phil. Mag. 17: 497, 698.
- C. Lindemann (1911). "Kinetic theory of melting". Phys. Zeitschr. 11: 609.
- ^ J. Frenkel (1946). Kinetic Theory of Liquids. Oxford University Press.
- D.B. Macleod (1936). "A note on the theory of viscosity of liquids". Trans. Far. Soc. 32: 872. doi:10.1039/tf9363200872.
- K.F. Herzfeld, M. Goeppert-Mayer (1934). "On the Theory of Fusion". Phys. Rev. 46: 995. doi:10.1103/PhysRev.46.995.
- M. Born (1939). "Thermodynamics of Crystals and Melting". J. Chem. Phys. 7: 591. doi:10.1063/1.1750497.
- M. Born (1940). "On the stability of crystal lattices I". Proc. Camb. Phil. Soc. 36: 160.
- M. Born, R. Furth (1940). "The stability of crystal lattices III". Proc. Camb. Phil. Soc. 36: 454.
- R.D. Misra (1941). "On the stability of crystal lattices II". Proc. Camb. Phil. Soc. 36: 173.
- R. Furth (1941). "The stability of crystal lattices". Proc. Camb. Phil. Soc. 37: 34.
- M. Born (1942). "On the stability of crystal lattices IX. Covariant theory of lattice deformations and the stability of some hexagonal lattices". Proc. Camb. Phil. Soc. 38: 82.
- M. Born, R.D. Misra (1943). "On the stability of crystal lattices IV". Proc. Camb. Phil. Soc. 36: 466.
- M. Born, M. Bradburn (1943). "The thermodynamics of crystal lattices". Proc. Camb. Phil. Soc. 39: 104.
- M. Bradburn (1943). "The thermodynamics of crystal lattices". Proc. Camb. Phil. Soc. 39: 113.
- M. Born (1944). "The thermodynamics of crystal lattices". Proc. Camb. Phil. Soc. 39: 100.
- M.M. Gow (1944). "The thermodynamics of crystal lattices". Proc. Camb. Phil. Soc. 40: 151.
-
W. Kauzmann, H. Eyring (1940). J. Am. Chem. Soc. 52: 3113.
{{cite journal}}: Missing or empty|title=(help) - ^ J. Frenkel (1937). "On the liquid state and the theory of fusion". Trans. Far. Soc. 33: 58. doi:10.1039/TF9373300058.
-
G.W. Scherer (1992). Relaxation in Glass and Composites. Krieger.
{{cite book}}: Unknown parameter|ibsn=ignored (help) - R. Zwanzig, R.D. Mountain (1965). "High-Frequency Elastic Moduli of Simple Fluids". J. Chem. Phys. 43: 4464. doi:10.1063/1.1696718.
- P.A. Fleury (1976). "Central-Peak Dynamics at the Ferroelectric Transition in Lead Germanate". Phys. Rev. Lett. 37: 1088. doi:10.1103/PhysRevLett.37.1088.
-
P.A. Fleury (1983). "Light Scattering Near Phase Transitions".
{{cite journal}}: Cite journal requires|journal=(help) -
P.A. Fleury (1974). "Anharmonic Lattices, Structural Transitions and Melting".
{{cite journal}}: Cite journal requires|journal=(help) -
G. Tammann (1925). "States of Aggregation".
{{cite journal}}: Cite journal requires|journal=(help) - G. Hagg (1935). "The Vitreous State". J. Chem. Phys. 3: 42.
- B.E. Warren (1937). "X-Ray Determination of the Structure of Liquids and Glasses". J. Appl. Phys. 8: 645. doi:10.1063/1.1710241.
- B.E. Warren (1938). "The Basic Principles Involved in the Glassy State". J. Appl. Phys. 13: 602.
-
W.H. Zachariasen (1932). J. Am. Chem. Soc. 54: 3841. doi:10.1021/ja01349a006.
{{cite journal}}: Missing or empty|title=(help) -
A.R. Cooper (1976). Phys. Chem. Glasses. 17: 38.
{{cite journal}}: Missing or empty|title=(help) -
A.R. Cooper (1975). Phys. Chem. Glasses. 19: 60.
{{cite journal}}: Missing or empty|title=(help) -
G.O. Jones (1948). Rep. Prog. Phys. 12: 133.
{{cite journal}}: Missing or empty|title=(help) - W. Kauzmann (1948). "The Nature of the Glassy State and the Behavior of Liquids at Low Temperatures". Chem. Rev. 43: 219. doi:10.1021/cr60135a002.
- R.O. Davies, G.O. Jones (1953). "The Irreversible Approach to Equilibrium in Glasses". Proc. Roy. Soc. London A. 217: 26.
- R.O. Davies, G.O. Jones (1953). "Thermodynamic and kinetic properties of glasses". Adv. Phys. 2: 370. doi:10.1080/00018735300101252.
-
I. Prigogine, R. Defay (1954). "Chemical Thermodynamics".
{{cite journal}}: Cite journal requires|journal=(help) - J. Olmsted, G.M. Williams (1994). Chemistry: The Molecular Science. Jones & Bartlett. ISBN 0815184506.
-
W.A. Weyl, B.C. Marboe (1962). "The Constitution of Glasses: A Dynamic Intepretation".
{{cite journal}}: Cite journal requires|journal=(help) -
D.R. Uhlmann (1972). J. Non-Cryst. Sol. 1: 337.
{{cite journal}}: Missing or empty|title=(help) -
G.M. Bartenev (1970). "Structure & Mechanical Properties of Inorganic Glasses". Walters-Noordhoff. ISBN 9001054501.
{{cite journal}}: Cite journal requires|journal=(help) -
C.A. Angell, J.H.R. Clarke, I.V. Woodcock (1981). "Interaction Potentials and Glass Formation: A Survey of Computer Experiments". Adv. Chem. Phys. 48: 397. doi:10.1002/9780470142684.ch5.
{{cite journal}}: CS1 maint: multiple names: authors list (link) - C.A. Angell (1981). "The Glass Transition: Comparison of Computer Simulation and Laboratory Studies". Trans. N.Y. Acad. Sci. 371: 136.
- D. Frenkel, J.P. McTague (1980). "Computer Simulations of Freezing and Supercooled Liquids". Ann. Rev. Phys. Chem. 31: 491. doi:10.1146/annurev.pc.31.100180.002423.
- J.P. Hansen and D. Levesque (1970). "Fluid-Solid Phase Transition of a Hard-Sphere Bose System". Phys. Rev. A. 2: 2514.
-
D. Levesque; et al. (1970). "Computer "Experiments" on Classical Fluids. III. Time-Dependent Self-Correlation Functions". Phys. Rev. A. 2: 2514.
{{cite journal}}: Explicit use of et al. in:|author=(help) -
D. Levesque; et al. (1973). "Computer "Experiments" on Classical Fluids. IV. Transport Properties and Time-Correlation Functions of the Lennard-Jones Liquid near Its Triple Point". Phys. Rev. A. 7: 1690.
{{cite journal}}: Explicit use of et al. in:|author=(help) -
D. Levesque; et al. (1979). "Liquid-glass transition, a free-volume approach". Phys. Rev. B. 20: 1077.
{{cite journal}}: Explicit use of et al. in:|author=(help) - G. Jacucci, I.R McDonald (1980). "Shear waves in liquid metals". Molec. Phys. 39: 515. doi:10.1080/00268978000100411.
- G.S. Grest (1977). "Kinetic theory of transport coefficients near Tc in a dynamic spherical model". Phys. Rev. B. 15: 2753.
- M.H. Cohen and G.S. Grest (1980). "Origin of Low-Temperature Tunneling States in Glasses". Phys. Rev. Lett. 45: 1271. doi:10.1103/PhysRevLett.45.1271.
- M.H. Cohen and G.S. Grest (1980). "Liquid-glass transition: Dependence of the glass transition on heating and cooling rates". Phys. Rev. B. 21: 4113.
-
G.S. Grest, S.R. Nagel, R. Rahman (1980). "Longitudinal and Transverse Excitations in a Glass". Phys. Rev. Lett. 49: 1271.
{{cite journal}}: CS1 maint: multiple names: authors list (link) -
W.P. Mason; et al. (1948). "Mechanical Properties of Long Chain Molecule Liquids at Ultrasonic Frequencies". Phys. Rev. 73: 1074. doi:10.1103/PhysRev.73.1074.
{{cite journal}}: Explicit use of et al. in:|author=(help) - W.P. Mason, H.J. McSkimmin (1949). "Measurement of Shear Elasticity and Viscosity of Liquids by Means of Ultrasonic Shear Waves". J. Acoust. Soc. Am. 21: 58. doi:10.1121/1.1917040.
- T.A. Litovits (1959). "Ultrasonic Spectroscopy in Liquids". J. Acoust. Soc. Am. 31: 681.
-
T.A. Litovitz; et al. (1954). "Ultrasonic Relaxation and Its Relation to Structure in Viscous Liquids". J. Acoust. Soc. Am. 26: 566. doi:10.1121/1.1907376.
{{cite journal}}: Explicit use of et al. in:|author=(help) - W.M. Madigosky, T.A. Litovitz (1960). "Mean Free Path and Ultrasonic Vibrational Relaxation in Liquids". J. Acoust. Soc. Am. 32: 928. doi:10.1121/1.1936510.
- J.T. Lamacchia (1967). "Brillouin Scattering in Viscoelastic Liquids (Ph.D. Thesis)". Dissertation Abstracts International. 27–09: 3218. Bibcode:1966PhDT........50L.
-
D. Pinnow; et al. (1967). "On the Relation of the Intensity of Scattered Light to the Viscoelastic Properties of Liquids and Glasses". J. Acoust. Soc. Am. 41: 1601. doi:10.1121/1.2143676.
{{cite journal}}: Explicit use of et al. in:|author=(help) -
C.J. Montrose; et al. (1968). "Brillouin Scattering and Relaxation in Liquids". J. Acoust. Soc. Am. 43: 117. doi:10.1121/1.1910741.
{{cite journal}}: Explicit use of et al. in:|author=(help) - I.L. Fabelinskii (1957). "Theory of Light Scattering in Liquids and Solids". Adv. Phys. Sci. (USSR). 63: 474.
-
S.P. Chen; et al. (1985). "Orientational ordering of local shear stresses in liquids: A phase transition?". J. Non-Cryst. Sol. 75: 449. doi:10.1016/0022-3093(85)90256-X.
{{cite journal}}: Explicit use of et al. in:|author=(help) - H.M. Rosenburg (1963). Low Temperature Solid State Physics. Clarendon Press.
- C.J. Kittel (1946). "Ultrasonic Propagation in Liquids. II. Theoretical Study of the Free Volume Model of the Liquid State". J. Chem. Phys. 14: 614. doi:10.1063/1.1724073.
- C.J. Kittel (1949). "Interpretation of the Thermal Conductivity of Glasses". Phys. Rev. 75: 972. doi:10.1103/PhysRev.75.972.
- ^ P.G. Klemens (1951). "The Thermal Conductivity of Dielectric Solids at Low Temperatures". Proc. Roy. Soc. London. 4208: 108.
- G.K. Chan, R.E Jones (1962). "Low-Temperature Thermal Conductivity of Amorphous Solids". Phys. Rev. 126: 2055.
-
I. Pomeranchuk (1941). J. Phys. (USSR). 4: 357.
{{cite journal}}: Missing or empty|title=(help) - R.C. Zeller, R.O. Pohl (1971). "Thermal Conductivity and Specific Heat of Non-crystalline Solids". Phys. Rev. B. 4: 2029.
- W.F. Love (1973). "Low-Temperature Thermal Brillouin Scattering in Fused Silica and Borosilicate Glass". Phys. Rev. Lett. 31: 822. doi:10.1103/PhysRevLett.31.822.
- M.P. Zaitlin, M.C. Anderson (1975). "Phonon thermal transport in noncrystalline materials". Phys. Rev. B. 12: 4475.
-
M.P. Zaitlin, L.M. Scherr, M.C. Anderson (1975). "Boundary scattering of phonons in noncrystalline materials". Phys. Rev. B. 12: 4487.
{{cite journal}}: CS1 maint: multiple names: authors list (link) -
C.L. Jr Reynolds (1979). J. Non-Cryst. Sol. 39: 371.
{{cite journal}}: Missing or empty|title=(help) - N.F. Mott (1934). "The Resistance of Liquid Metals". Proc. Roy. Soc. London A. 146: 465.
-
C. Lindemann (1911). Phys. Zeitschr. 11: 609.
{{cite journal}}: Missing or empty|title=(help) -
W. Jr Klement, I.H. Willens, P. Duwez (1960). "Non-crystalline Structure in Solidified Gold–Silicon Alloys". Nature. 187: 359. doi:10.1038/187869b0.
{{cite journal}}: CS1 maint: multiple names: authors list (link) -
W. Klement, I.H. Willens, P. Duwez (1960). "Continuous Series of Metastable Solid Solutions in Silver-Copper Alloys". J. Appl. Phys. 31: 1136.
{{cite journal}}: CS1 maint: multiple names: authors list (link) -
W. Klement, I.H. Willens, P. Duwez (1960). "Metastable Electron Compound in Ag-Ge Alloys". J. Appl. Phys. 31: 1137.
{{cite journal}}: CS1 maint: multiple names: authors list (link) -
H.A. Davies (1976). Phys. Chem. Glasses. 17: 159.
{{cite journal}}: Missing or empty|title=(help) -
H.A. Davies (1979). Rev. De Chim. Min. 16: 349.
{{cite journal}}: Missing or empty|title=(help) - P. Chaudhari, P. D. Turnbull (1978). "Structure and Properties of Metallic Glasses". Science. 199: 11. doi:10.1126/science.199.4324.11. PMID 17841932.
-
J.S. Chen (1980). Rep. Progr. Phys. 43: 353.
{{cite journal}}: Missing or empty|title=(help) -
J.J. Gilman (1978). Science. 199: 11.
{{cite journal}}: Missing or empty|title=(help) - ^ M. Jonson, S.M. Girvin (1979). "Electron-Phonon Dynamics and Transport Anomalies in Random Metal Alloys". Phys. Rev. Lett. 43: 1447. doi:10.1103/PhysRevLett.43.1447.
-
M. Jonson, S.M. Girvin (1980). Phys. Rev. B. 22: 3283.
{{cite journal}}: Missing or empty|title=(help) -
D. Turnbull (1974). J. Phys. C. 35: 1.
{{cite journal}}: Missing or empty|title=(help) - H.S. Chen, B.K. Park (1973). "Role of chemical bonding in metallic glasses". Acta. Met. 21: 395. doi:10.1016/0001-6160(73)90196-X.
- R. Wang, D. Merz (1977). "Polymorphic bonding and thermal stability of elemental noncrystalline solids". Phys. Stat. Sol. A. 39: 697.
Further reading
- A. Pais (1982). Subtle is the Lord: The Science and the Life of Albert Einstein. Oxford University Press.
- L.D. Landau, L.D., E.M. Lifshitz (1986). Theory of Elasticity. Oxford University Press.
{{cite book}}: CS1 maint: multiple names: authors list (link) - G. Srivastriva (1990). The Physics of Phonons. Taylor & Francis.
- R. Zallen (1998). The Physics of Amourphous Solids. Wiley Interscience.
- S.R. Elliot (1990). The Physics of Amorphous Materials (2nd ed.). Longman.
- N. Cusack (1987). The Physics of Structurally Disordered Matter: An Introduction. IOP Publishing.
- N.H. March (ed), R.A. Street (ed), M.P. Tosi (ed) (1985). Amorphous Solids and the Liquid State. Springer.
{{cite book}}:|author=has generic name (help)CS1 maint: multiple names: authors list (link) - D.A. Adler (ed), B.B. Schwartz (ed), M.C. Steele (ed) (1985). Physical Properties of Amorphous Materials. Springer.
{{cite book}}:|author=has generic name (help)CS1 maint: multiple names: authors list (link) - A. Inoue (ed), K. Hasimoto (ed) (1985). Amorphous and Nanocrystalline Materials. Springer.
{{cite book}}:|author=has generic name (help) - J.H. Crawford (ed), L.M. Slifkin (ed) (1975). Point Defect in Solids. Plenum Press.
{{cite book}}:|author=has generic name (help) - J.P. Hirth, J. Lothe (1992). Theory of Dislocations (2nd ed.). Krieger.
- N.D. Mermin (1979). "Topological Theory of Defects". Rev. Mod. Phys. 1: 591.
- J.W. Christian (1975). Theory of Phase Transformations in Metals and Alloys. Permagon Press.
- A.G. Khachaturyan (1983). Theory of Structural Transformations in Solids. Dover Publications.
- A.P. French (1971). Vibrations and Waves.
- Y. Katznelson (1976). An Introduction to Harmonic Analysis. Dover Publications.
- J. Fourier (2003) . The Analytical Theory of Heat. Dover Publications. ISBN 0486495310.
{{cite book}}: Unknown parameter|other=ignored (|others=suggested) (help)
External links
- American Ceramic Society
- Angell, C.A.: Collected Works
- Scherer, G.W.: Collected Works
- MacKenzie, J.D.: Curriculum Vitae
- Journal of Non-Crystalline Solids
- Physical Properties of Glass



 is defined as:
is defined as:
